超深层高压油气藏天然气偏差系数计算新模型

杨鹏程; 薛浩楠; 李升; 陈科杉

doi:10.11911/syztjs.2023112

超深层高压油气藏天然气偏差系数计算新模型

1.
中国石油集团西部钻探工程有限公司井下作业公司，新疆克拉玛依 834000
2.
中国石油大学（华东）石油工程学院，山东青岛 266580

详细信息

作者简介:
杨鹏程（1980—），男，辽宁海城人，2006年毕业于中国石油大学（华东）石油工程专业，工程师，主要从事储层改造工作。E-mail：38850115@qq.com

中图分类号: TE311⁺.1
计量
- 文章访问数: 97
- HTML全文浏览量: 17
- PDF下载量: 33
出版历程
- 收稿日期: 2023-03-10
- 修回日期: 2023-10-31
- 网络出版日期: 2023-12-06
- 刊出日期: 2023-11-24

A New Model for Calculating Deviation Factor of Natural Gas in Ultra-Deep Oil and Gas Reservoirs under High Pressure

1.
Downhole Operation Company, CNPC Xibu Drilling Engineering Company Limited, Karamay, Xinjiang, 834000, China
2.
School of Petroleum Engineering, China University of Petroleum (East China), Qingdao, Shandong, 266580, China

摘要

摘要:
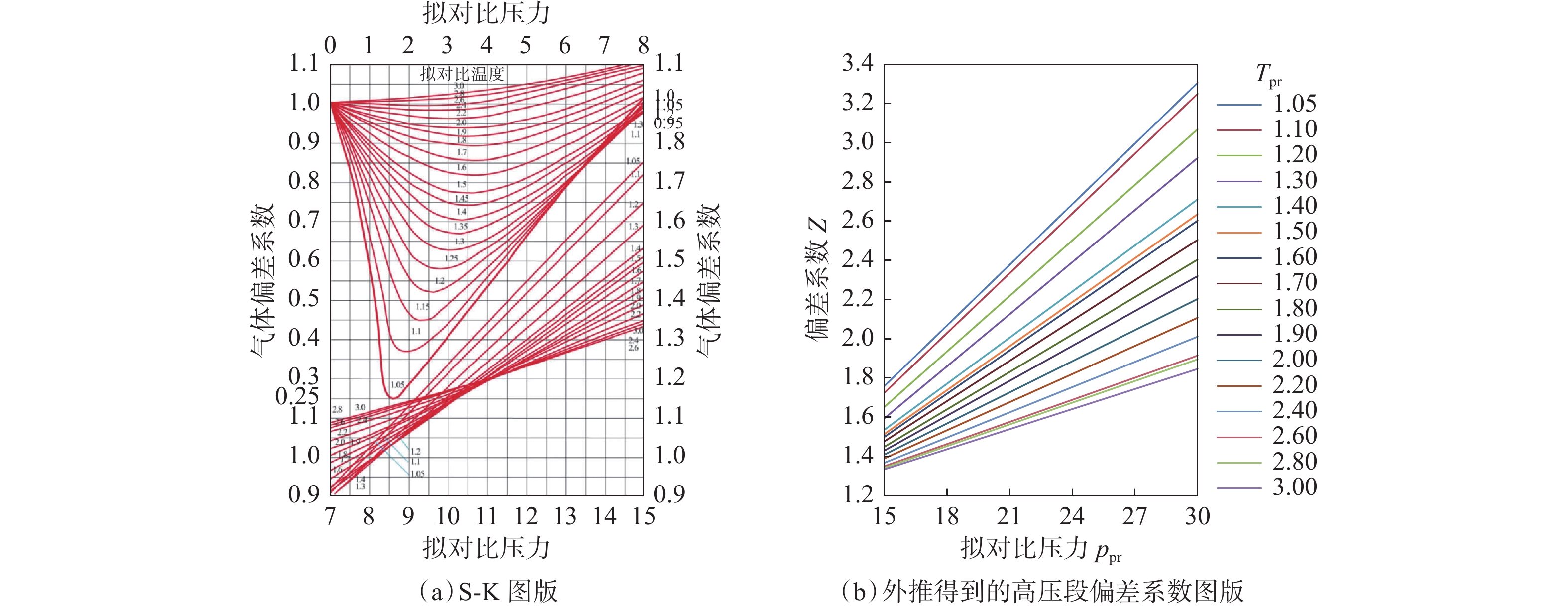
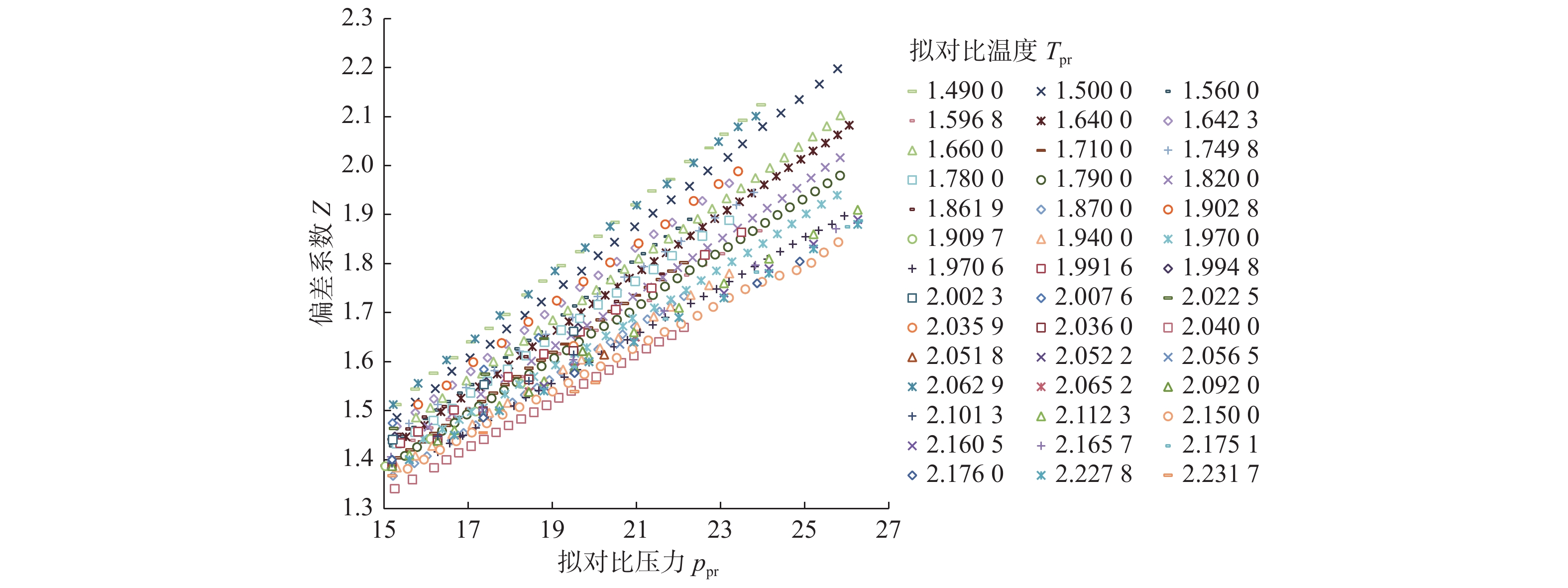
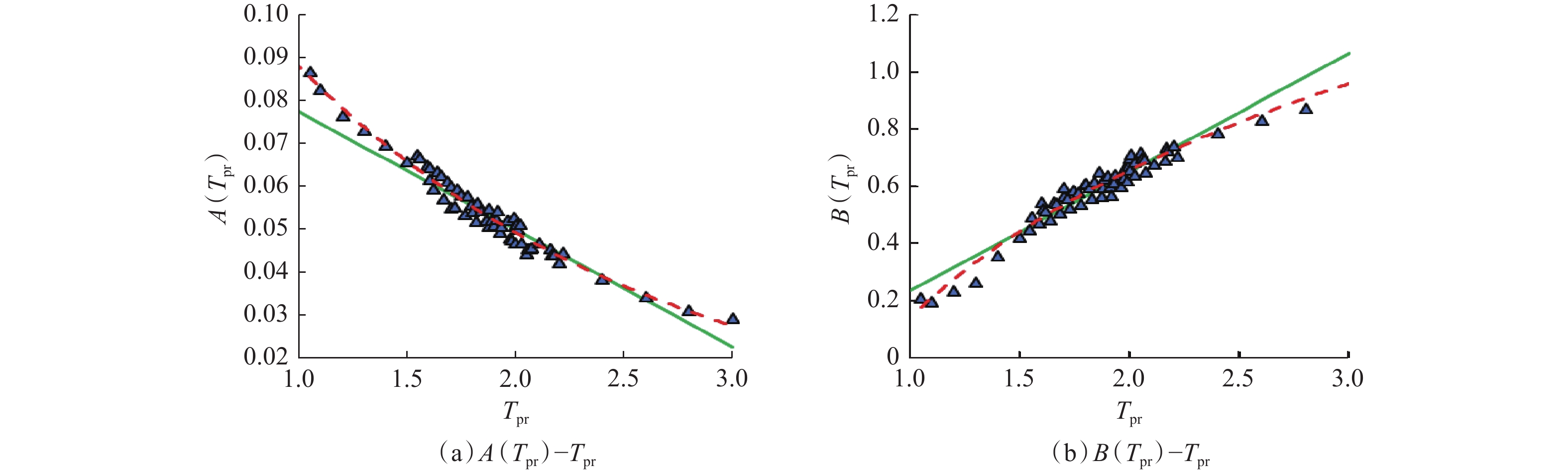
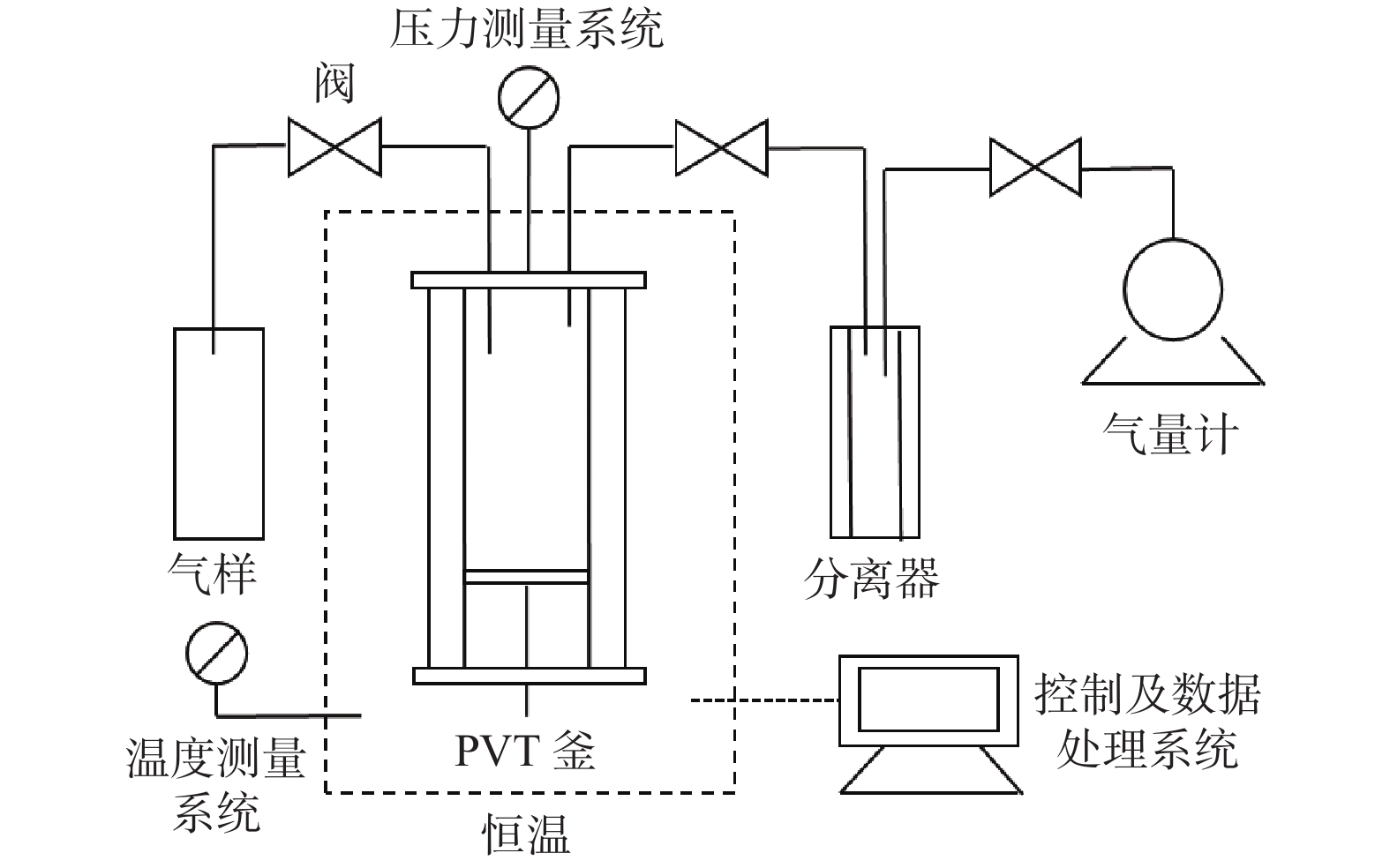
为适应我国深层、超深层高压油气藏钻采需要，利用高温高压PVT特性测量系统，开展了高压条件下不同组分天然气样品的PVT特性测量试验，试验结果表明，高压下天然气偏差系数随压力升高大致呈线性增大，随温度升高而减小，但总体差别较小。基于1443组Standing-Katz图版拟合数据、试验测量数据和公共试验数据，建立了大温度压力范围的天然气偏差系数试验数据库。采用多元非线性拟合数值方法，对现有模型进行改进，建立了计算超深层高压油气藏天然气偏差系数的新模型。将该模型与常用的HY法、DPR法、LXF法等方法进行了对比，误差分析表明，该模型在高压段的相对误差在2%以内，计算精度高于HY法、DPR法、LXF法等方法，满足工程要求，可以为超深层高压油气藏安全高效钻采提供指导与帮助。
- 超深层高压油气藏 /
- 天然气偏差系数 /
- 数据库 /
- 计算模型 /
- Standing-Katz图版 /
- 误差
Abstract:
In order to meet the drilling and production requirements of deep and ultra-deep oil and gas reservoirs under high pressure in China, PVT characteristic measurement experiments of natural gas samples with different components under high-pressure conditions were carried out by using high-temperature and high-pressure PVT characteristic measurement system. The experiments show that the deviation factor of natural gas under high pressure increases linearly with the increase in pressure and decreases with the increase in temperature, but the overall difference is small. At the same time, an experimental database of the deviation factor of natural gas in a large temperature and pressure range is established based on 1443 sets of data including Standing-Katz chart fitting data, experimental measurement data, and public experimental data. Through the numerical method of multivariate nonlinear fitting, the existing models are improved, and a new model for calculating the deviation factor of natural gas of ultra-deep oil and gas reservoirs under high pressure is established. The predicted results of the model are compared with those of HY, DPR, LXF, and other common methods. The error analysis shows that the relative error of the model is less than 2% in the high-pressure section, and its calculation accuracy is higher than that of HY, DPR, LXF, and other methods, which meets the practical needs of engineering and can provide guidance and support for safe and efficient drilling and production of ultra-deep oil and gas reservoirs under high pressure.
- ultra-deep reservoirs under high pressure /
- deviation factor of natural gas /
- database /
- calculation model /
- Standing-Katz chart /
- error

HTML全文

图 1 超高压全可视PVT测试系统结构示意

Figure 1. Structure of ultra-high pressure and fully visual PVT test system

下载: 全尺寸图片幻灯片

图 2 试验条件下天然气样品1和样品2偏差系数的分布规律

Figure 2. Distribution law of deviation factor of natural gas of sample 1 and sample 2 under experimental conditions

下载: 全尺寸图片幻灯片

图 3 S-K图版和外推得到的高压段偏差系数图版

Figure 3. S-K chart and extrapolated deviation factor relationship chart of high-pressure section

下载: 全尺寸图片幻灯片

图 4 高压下天然气偏差系数试验数据库

Figure 4. Experimental database of deviation factor of natural gas under high pressure

下载: 全尺寸图片幻灯片

图 5 A(T_pr)和B(T_pr)分别与拟对比温度T_pr的关系

Figure 5. Relationship of A(T_pr) and B(T_pr) with temperature T_pr respectively

下载: 全尺寸图片幻灯片

图 6 新模型以及不同计算方法与实测天然气偏差系数对比

Figure 6. Comparison of deviation factor of natural gas of new model and different calculation methods with measured data

下载: 全尺寸图片幻灯片

表 1 样品1和样品2天然气组分分析结果

Table 1 Analysis of natural gas components of sample 1 and sample 2

组分	含量，%
组分	样品1	样品2
二氧化碳	1.782	0.53
氮气	0.302	0.66
甲烷	81.493	95.04
乙烷	12.042	2.46
丙烷	2.606	0.44
异丁烷	0.445	0.11
正丁烷	0.518	0.12
异戊烷	0.162	0.06
正戊烷	0.132	0.05
己烷	0.150	0.10
庚烷	0.096	0.10
辛烷	0.071	0.12
壬烷	0.029	0.06
癸烷	0.028	0.03
十一烷及以上	0.145	0.11

下载: 导出CSV

表 2 常用天然气偏差系数计算方法

Table 2 Commonly used calculation method for deviation factor of natural gas

计算方法	模型形式	适用范围
Beggs-Brill法^[26]	$Z = A\left( {1 - A} \right)\exp \left( { - B} \right) + Cp_{ {\text{pr} } }^D$	0≤p_pr≤10.0； 1.2≤T_pr≤2.4
HY法^[19]	$Z = \left[ {\dfrac{ {0.061\;25{p_{ {\text{pr} } } }t} }{ { {\rho _{ {\text{pr} } } } } } } \right]\exp \left[ { - 1.2{ {(1 - t)}^2} } \right]$	0.1≤p_pr≤24.0； 1.2≤T_pr≤3.0
DPR法^[20]	${\begin{gathered} Z = 1 + \left( { {A_1} + {A_2}/{T_{ {\text{pr} } } } + {A_3}/T_{ {\text{pr} } }^3} \right){\rho _{ {\text{pr} } } } +\\ \left( { {A_4} + {A_5}/{T_{ {\text{pr} } } } } \right)\rho _{ {\text{pr} } }^2 + {A_5}{A_6}\rho _{ {\text{pr} } }^5/{T_{ {\text{pr} } } }+ \\ {A_7}\left( {1 + {A_8}\rho _{ {\text{pr} } }^2} \right)\rho _{ {\text{pr} } }^2\exp \left( { - {A_8}\rho _{ {\text{pr} } }^2} \right)/T_{ {\text{pr} } }^3 \end{gathered} }$	0.2≤p_pr≤30.0； 1.05≤T_pr≤3.00
DAK法^[21]	$\begin{gathered} Z = 1 + \left( { {A_1} + {A_2}/{T_{ {\text{pr} } } } + {A_3}/T_{ {\text{pr} } }^3 + {A_4}/T_{ {\text{pr} } }^4 + {A_5}/T_{ {\text{pr} } }^5} \right){\rho _{ {\text{pr} } } } + \\ \left( { {A_6} + {A_7}/{T_{ {\text{pr} } } } + {A_8}/T_{ {\text{pr} } }^2} \right)\rho _{ {\text{pr} } }^2 - {A_9}\left( { {A_7}/{T_{ {\text{pr} } } } + {A_8}/T_{ {\text{pr} } }^2} \right)\rho _{ {\text{pr} } }^5 +\\ {A_{10} }\left( {1 + {A_{11} }\rho _{ {\text{pr} } }^2} \right)\left( {\rho _{ {\text{pr} } }^2/T_{ {\text{pr} } }^3} \right)\exp \left( { - {A_{11} }\rho _{ {\text{pr} } }^2} \right) \end{gathered}$	0.2≤p_pr＜30.0； 1＜T_pr≤3
E.Heidaryan等的方法^[27]	$Z = \dfrac{{{A_1} + {A_2}\ln \left( {{p_{{\text{pr}}}}} \right) + {A_3}{{\left( {\ln {p_{{\text{pr}}}}} \right)}^2} + {A_4}{{\left( {\ln {p_{{\text{pr}}}}} \right)}^3} + \dfrac{{{A_5}}}{{{T_{{\text{pr}}}}}} + \dfrac{{{A_6}}}{{T_{{\text{pr}}}^2}}}}{{1 + {A_7}\ln \left( {{p_{{\text{pr}}}}} \right) + {A_8}{{\left( {\ln {p_{{\text{pr}}}}} \right)}^2} + \dfrac{{{A_9}}}{{{T_{{\text{pr}}}}}} + \dfrac{{{A_{10}}}}{{T_{{\text{pr}}}^2}}}}$	0.2≤p_pr≤15.0； 1.2≤T_pr≤3.0
LXF法^[11]	$Z = {x_{ {\text{F1} } } }{p_{ {\text{pr} } } } + {x_{ {\text{F2} } } }$	0≤p_pr≤30.0； 1.05≤T_pr≤3.00
ZGD法^[12]	$\begin{gathered} Z = \left( { {A_1}T_{{\rm{pr}}}^4 + {A_2}T_{{\rm{pr}}}^3 + {A_3}T_{{\rm{pr}}}^2} \right.\left. { + {A_4}{T_{{\rm{pr}}} } + {A_5} } \right){p_{{\rm{pr}}} }+ \\ \left( { {A_6}T_{{\rm{pr}}}^4 + {A_7}T_{{\rm{pr}}}^3} \right.{A_8}{T_{{\rm{pr}}} }^2\left. { + {A_9}{T_{{\rm{pr}}} } + {A_{10} } } \right) \end{gathered}$	8.0≤p_pr＜30.0； 1.05≤T_pr＜3.00
W-Z-G法^[23]	$Z = ap_{ {\text{pr} } }^2 + b{p_{ {\text{pr} } } } + c$	15.0≤p_pr≤30.0； 1.6≤T_pr≤2.4

下载: 导出CSV

表 3 高压下天然气偏差系数试验数据库

Table 3 Experimental database of deviation factor of natural gas under high pressure

数据来源	p/MPa	T/K	数据点数
文献[33]	70.00～100.00	323.15～413.15	60
文献[34]	70.00～110.00	359.79～384.85	20
文献[7]	70.00～122.88	409.85～429.85	33
文献[35]	70.00～118.89	313.20～407.20	320
文献[36]	70.00～110.00	313.15～404.55	60
文献[37]	70.00～114.68	399.25～437.65	26
本文试验数据	0～120.00	383.15～423.15	284
总计			803

下载: 导出CSV

表 4 新模型计算值与实测值相对误差

Table 4 Relative error between calculated and experimental results of new model

本文试验数据						文献[39]试验数据
T_pr=1.71		T_pr=1.90		T_pr=2.18		T_pr=2.13
p_pr	相对误差，%	p_pr	相对误差，%	p_pr	相对误差，%	p_pr	相对误差，%
22.13	1.66	23.42	0.77	26.28	0.15	22.49	1.03
21.57	1.77	22.95	0.37	25.97	0.06	21.96	0.87
21.28	1.75	22.36	0.50	24.89	0.06	21.32	0.65
20.71	1.74	21.68	0.55	23.81	0.12	20.90	0.51
20.15	1.79	21.05	0.57	22.73	0.02	20.58	0.38
19.57	1.80	20.37	0.37	21.64	0.21	20.26	0.27
19.02	1.91	19.74	0.20	20.56	0.02	19.83	0.08
18.45	1.90	19.11	0.46	19.48	0.11	19.30	0.08
17.88	1.97	18.43	0.48	18.40	0.07	18.83	0.23
17.32	1.69	17.79	0.61	17.31	0.08	18.51	0.37
16.75	1.86	17.12	0.45	16.23	0.12	18.12	0.53
16.19	1.84	16.48	0.46	15.15	0.11
15.62	1.96	15.81	0.30			15.54	1.69
15.03	1.61					15.20	1.90
平均	1.80		0.47		0.09		0.78

下载: 导出CSV

表 5 不同计算方法平均相对误差对比

Table 5 Comparison of average relative errors of different calculation methods

拟对比温度	平均相对误差，%
拟对比温度	HY法	DPR法	LXF法	ZGD法	W-Z-G法	新模型
T_pr=1.71	22.23	22.02	2.89	3.39	19.46	1.80
T_pr=1.90	23.38	23.73	0.67	0.51	8.38	0.47
T_pr=2.13^[39]	17.00	17.47	0.80	0.83	1.33	0.78
T_pr=2.18	21.86	22.04	0.99	1.09	3.22	0.09

下载: 导出CSV

参考文献(39)

[1]	李月清. 超深层油气资源接续潜力巨大[J]. 中国石油企业,2023(7):50–51. LI Yueqing. Great potential for hydrocarbon resource succession in ultra-deep depth[J]. China Petroleum Enterprise, 2023(7): 50–51.
[2]	何立成,唐波. 准噶尔盆地超深井钻井技术现状与发展建议[J]. 石油钻探技术,2022,50(5):1–8. doi: 10.11911/syztjs.2022092 HE Licheng, TANG Bo. The up to date technologies of ultra-deep well drilling in Junggar basin and suggestions for further improvements[J]. Petroleum Drilling Techniques, 2022, 50(5): 1–8. doi: 10.11911/syztjs.2022092
[3]	孙贺东,曹雯,李君,等. 提升超深层超高压气藏动态储量评价可靠性的新方法:物质平衡实用化分析方法[J]. 天然气工业,2020,40(7):49–56. doi: 10.3787/j.issn.1000-0976.2020.07.006 SUN Hedong, CAO Wen, LI Jun, et al. A material balance based practical analysis method to improve the dynamic reserve evaluation reliability of ultra-deep gas reservoirs with ultra-high pressure[J]. Natural Gas Industry, 2020, 40(7): 49–56. doi: 10.3787/j.issn.1000-0976.2020.07.006
[4]	肖香姣,闫柯乐,王海应,等. 一种预测超高压气藏压缩因子的新方法[J]. 天然气工业,2012,32(10):42–46. doi: 10.3787/j.issn.1000-0976.2012.10.010 XIAO Xiangjiao, YAN Kele, WANG Haiying, et al. A new approach to predicting the compressibility factor of ultra high-pressure gas reservoirs[J]. Natural Gas Industry, 2012, 32(10): 42–46. doi: 10.3787/j.issn.1000-0976.2012.10.010
[5]	陈丽群,孙雷,张立强,等. 超高压气藏天然气偏差因子计算方法分析[J]. 油气藏评价与开发,2012,2(6):24–27. doi: 10.13809/j.cnki.cn32-1825/te.2012.06.005 CHEN Liqun, SUN Lei, ZHANG Liqiang, et al. Study on natural gas Z-factor computing methods of overpressured gas reservoir[J]. Reservoir Evaluation and Development, 2012, 2(6): 24–27. doi: 10.13809/j.cnki.cn32-1825/te.2012.06.005
[6]	刘传喜,刘华,王卫红. 基于物质平衡法确定超高压气藏动态储量的新方法[J]. 天然气工业,2009,29(12):68–70. doi: 10.3787/j.issn.1000-0976.2009.12.022 LIU Chuanxi, LIU Hua, WANG Weihong. New material balance-based methods of determining the dynamic reserves of ultra-high pressure gas reservoirs[J]. Natural Gas Industry, 2009, 29(12): 68–70. doi: 10.3787/j.issn.1000-0976.2009.12.022
[7]	于洋,谢南星,叶长青,等. 超高压气藏天然气偏差系数计算方法研究[J]. 天然气与石油,2021,39(5):66–73. doi: 10.3969/j.issn.1006-5539.2021.05.010 YU Yang, XIE Nanxing, YE Changqing, et al. Study on calculation method of deviation factor of natural gas in ultra-high pressure gas reservoir[J]. Natural Gas and Oil, 2021, 39(5): 66–73. doi: 10.3969/j.issn.1006-5539.2021.05.010
[8]	阳建平,肖香姣,张峰,等. 几种天然气偏差因子计算方法的适用性评价[J]. 天然气地球科学,2007,18(1):154–157. doi: 10.3969/j.issn.1672-1926.2007.01.031 YANG Jianping, XIAO Xiangjiao, ZHANG Feng, et al. Applicability estimation of four methods of calculating the deviation factor of natural gas[J]. Natural Gas Geoscience, 2007, 18(1): 154–157. doi: 10.3969/j.issn.1672-1926.2007.01.031
[9]	李旭成,曹建,梁静,等. 超高压气井储量计算方法研究[J]. 天然气技术与经济,2018,12(4):35–37. doi: 10.3969/j.issn.2095-1132.2018.04.010 LI Xucheng, CAO Jian, LIANG Jing, et al. Reserve calculation methods for extra-high-pressure gas wells[J]. Natural Gas Technology and Economy, 2018, 12(4): 35–37. doi: 10.3969/j.issn.2095-1132.2018.04.010
[10]	李相方,刚涛,庄湘琦,等. 高压天然气偏差系数的高精度解析模型[J]. 石油大学学报(自然科学版),2001,25(6):45–46. LI Xiangfang, GANG Tao, ZHUANG Xiangqi, et al. A analytic model with high precision for calculating compressibility factor of high-pressure gas[J]. Journal of the University of Petroleum, China, 2001, 25(6): 45–46.
[11]	李相方,任美鹏,胥珍珍,等. 高精度全压力全温度范围天然气偏差系数解析计算模型[J]. 石油钻采工艺,2010,32(6):57–62. doi: 10.3969/j.issn.1000-7393.2010.06.015 LI Xiangfang, REN Meipeng, XU Zhenzhen, et al. A high-precision and whole pressure temperature range analytical calculation model of natural gas Z-factor[J]. Oil Drilling & Production Technology, 2010, 32(6): 57–62. doi: 10.3969/j.issn.1000-7393.2010.06.015
[12]	张国东,李敏,柏冬岭. 高压超高压天然气偏差系数实用计算模型:LXF高压高精度天然气偏差系数解析模型的修正[J]. 天然气工业,2005,25(8):79–80. doi: 10.3321/j.issn:1000-0976.2005.08.025 ZHANG Guodong, LI Min, BAI Dongling. Practical calculating model of gas deviation factor with high and super-high pressure: Correction of the analytical model for the deviation coefficient of LXF high-pressure and high-precision natural gas[J]. Natural Gas Industry, 2005, 25(8): 79–80. doi: 10.3321/j.issn:1000-0976.2005.08.025
[13]	沈伟军,李熙喆,刘晓华,等. 异常高压气藏偏差系数计算方法筛选与推荐[J]. 科学技术与工程,2014,14(31):204–208. doi: 10.3969/j.issn.1671-1815.2014.31.038 SHEN Weijun, LI Xizhe, LIU Xiaohua, et al. The selections and recommendations of calculating deviation factor methods for abnormally pressured gas reservoirs[J]. Science Technology and Engineering, 2014, 14(31): 204–208. doi: 10.3969/j.issn.1671-1815.2014.31.038
[14]	董萌. 天然气压缩因子计算方法对比及应用[D]. 大庆: 东北石油大学, 2015. DONG Meng. Comparison methods of compression factor of natural gas and its application[D]. Daqing: Northeast Petroleum University, 2015.
[15]	王辉,李木盛,陈荟宇. 准确度更高的高含硫天然气压缩因子计算方法[J]. 石油与天然气化工,2022,51(6):50–60. doi: 10.3969/j.issn.1007-3426.2022.06.008 WANG Hui, LI Musheng, CHEN Huiyu. More accurate compression factor calculation method for high sulfur gas[J]. Chemical Engineering of Oil and Gas, 2022, 51(6): 50–60. doi: 10.3969/j.issn.1007-3426.2022.06.008
[16]	陈磊,耿耿,景红. 东海某气田天然气偏差系数实验及计算模型评价研究[J]. 海洋石油,2022,42(4):36–41. doi: 10.3969/j.issn.1008-2336.2022.04.036 CHEN Lei, GENG Geng, JING Hong. Study on gas deviation factor experiment and calculation model evaluation of a gas field in east China sea[J]. Offshore Oil, 2022, 42(4): 36–41. doi: 10.3969/j.issn.1008-2336.2022.04.036
[17]	STANDING M B, KATZ D L. Density of natural gases[J]. Transactions of the AIME, 1942, 146(1): 140–149. doi: 10.2118/942140-G
[18]	KATZ D L, CORNELL D, KOBAYASHI R, et al. Handbook of natural gas engineering[M]. New York: McGraw-Hill Book Company, Inc. , 1959: 106−107, 710−717.
[19]	HALL K R, YARBOROUGH L. A new equation of state for Z-factor calculations[J]. Oil and Gas Journal, 1973, 71(25): 82–92.
[20]	DRANCHUK P M, PURVIS R A, ROBINSON D B. Computer calculation of natural gas compressibility factors using the standing and Katz correlation[R]. PETSOC-73−112, 1973.
[21]	DRANCHUK P M, ABOU-KASSEM H. Calculation of Z factors for natural gases using equations of state[J]. Journal of Canadian Petroleum Technology, 1975, 14(3): 34–36.
[22]	王艺晨,叶继根,吴淑红. 基于对勾函数的新型天然气压缩因子计算关联式[J]. 石油与天然气化工,2021,50(5):38–43. doi: 10.3969/j.issn.1007-3426.2021.05.008 WANG Yichen, YE Jigen, WU Shuhong. A new correlation for calculating natural gas compressibility factor based on hook function[J]. Chemical Engineering of Oil and Gas, 2021, 50(5): 38–43. doi: 10.3969/j.issn.1007-3426.2021.05.008
[23]	颜雪,孙雷,周剑锋,等. 计算超高压气藏天然气偏差因子新方法[J]. 油气藏评价与开发,2015,5(1):26–29. doi: 10.3969/j.issn.2095-1426.2015.01.005 YAN Xue, SUN Lei, ZHOU Jianfeng, et al. New model of calculating natural gas Z-factor in the ultrahigh pressure gas reservoir[J]. Reservoir Evaluation and Development, 2015, 5(1): 26–29. doi: 10.3969/j.issn.2095-1426.2015.01.005
[24]	张立侠,郭春秋. 天然气偏差因子计算新方法[J]. 石油与天然气化工,2019,48(1):91–98. doi: 10.3969/j.issn.1007-3426.2019.01.016 ZHANG Lixia, GUO Chunqiu. A new method for determining the natural gas compressibility factor[J]. Chemical Engineering of Oil and Gas, 2019, 48(1): 91–98. doi: 10.3969/j.issn.1007-3426.2019.01.016
[25]	ORODU K B, OKORO E E, IJALAYE O K, et al. Gas compressibility factor explicit correlations for range of pseudo reduced temperature and pressure[J]. Flow Measurement and Instrumentation, 2019, 67: 176–185. doi: 10.1016/j.flowmeasinst.2019.05.003
[26]	BEGGS D H, BRILL J P. A study of two-phase flow in inclined pipes[J]. Journal of Petroleum Technology, 1973, 25(5): 607–617. doi: 10.2118/4007-PA
[27]	HEIDARYAN E, SALARABADI A, MOGHADASI J. A novel correlation approach for prediction of natural gas compressibility factor[J]. Journal of Natural Gas Chemistry, 2010, 19(2): 189–192. doi: 10.1016/S1003-9953(09)60050-5
[28]	MAHMOUD M. Development of a new correlation of gas compressibility factor (Z-factor) for high pressure gas reservoirs[J]. Journal of Energy Resources Technology, 2014, 136(1): 012903. doi: 10.1115/1.4025019
[29]	MOHAMADI-BAGHMOLAEI M, AZIN R, OSFOURI S, et al. Prediction of gas compressibility factor using intelligent models[J]. Natural Gas Industry B, 2015, 2(4): 283–294. doi: 10.1016/j.ngib.2015.09.001
[30]	CHAMKALANI A, ZENDEHBOUDI S, CHAMKALANI R, et al. Utilization of support vector machine to calculate gas compressibility factor[J]. Fluid Phase Equilibria, 2013, 358: 189–202. doi: 10.1016/j.fluid.2013.08.018
[31]	GHANEM A, GOUDA M F, ALHARTHY R D, et al. Predicting the compressibility factor of natural gas by using statistical modeling and neural network[J]. Energies, 2022, 15(5): 1807. doi: 10.3390/en15051807
[32]	李定军. 异常高压气藏天然气偏差系数的确定[J]. 石油实验地质,2012,34(6):656–658. doi: 10.11781/sysydz201206656 LI Dingjun. Determination of gas deviation factor for abnormal overpressure gas reservoir[J]. Petroleum Geology and Experiment, 2012, 34(6): 656–658. doi: 10.11781/sysydz201206656
[33]	郭绪强,王峰,陈光进. 特高压天然气压缩因子的实验测定[J]. 石油勘探与开发,1999,26(6):84–85. doi: 10.3321/j.issn:1000-0747.1999.06.025 GUO Xuqiang, WANG Feng, CHEN Guangjin. Measurement of the compressibility factor of natural gases at super high pressure[J]. Petroleum Exploration and Development, 1999, 26(6): 84–85. doi: 10.3321/j.issn:1000-0747.1999.06.025
[34]	冀光,夏静,罗凯,等. 超高压气藏气体偏差因子的求取方法[J]. 石油学报,2008,29(5):734–737. doi: 10.3321/j.issn:0253-2697.2008.05.018 JI Guang, XIA Jing, LUO Kai, et al. Determination of natural gas deviation factor of ultra-high pressured gas reservoir[J]. Acta Petrolei Sinica, 2008, 29(5): 734–737. doi: 10.3321/j.issn:0253-2697.2008.05.018
[35]	SUN Changyu, LIU Huang, YAN Kele, et al. Experiments and modeling of volumetric properties and phase behavior for condensate gas under ultra-high-pressure conditions[J]. Industrial & Engineering Chemistry Research, 2012, 51(19): 6916–6925.
[36]	HU Yongle, LUO Kai, LI Baozhu, et al. Phase behaviour and properties of HPHT gas condensate systems[R]. SPE 104423, 2006.
[37]	LIU Huang, WU Yiming, GUO Ping, et al. Compressibility factor measurement and simulation of five high-temperature ultra-high-pressure dry and wet gases[J]. Fluid Phase Equilibria, 2019, 500: 112256. doi: 10.1016/j.fluid.2019.112256
[38]	管虹翔,段国喜,齐桃,等. 一种新型天然气压缩因子数值计算方法[J]. 特种油气藏,2011,18(2):85–88. doi: 10.3969/j.issn.1006-6535.2011.02.024 GUAN Hongxiang, DUAN Guoxi, QI Tao, et al. A new computing method of gas compressibility factor[J]. Special Oil & Gas Reservoirs, 2011, 18(2): 85–88. doi: 10.3969/j.issn.1006-6535.2011.02.024
[39]	YAN Kele, LIU Huang, SUN Changyu, et al. Measurement and calculation of gas compressibility factor for condensate gas and natural gas under pressure up to 116 MPa[J]. The Journal of Chemical Thermodynamics, 2013, 63: 38–43. doi: 10.1016/j.jct.2013.03.025

施引文献(5)

期刊类型引用(4)

1.	耿学礼，郑晓斌，苏延辉，敬倩，史斌，李建. 沁南区域煤层气水平井瓜尔胶钻井液技术. 石油钻探技术. 2023(01): 34-39 . 本站查看
2.	梁龙军，陈捷，颜智华，高为，易旺，胡海洋. 六盘水煤田大倾角地层煤层气L型水平井钻完井技术. 断块油气田. 2023(04): 616-623 . 百度学术
3.	陈天，易远元，李甜甜，兰天庆. 中国煤层气勘探开发现状及关键技术展望. 现代化工. 2023(09): 6-10 . 百度学术
4.	张冲. 煤矿区煤层气水平对接井轨迹控制与完井技术研究分析. 中国石油和化工标准与质量. 2022(09): 177-179 . 百度学术