南川页岩气田压裂工艺改进及应用

周成香; 房大志; 王旭; 李成赢; 王志峰

doi:10.11911/syztjs.2025010

南川页岩气田压裂工艺改进及应用

中石化重庆页岩气有限公司，重庆 408400

基金项目: 中国石化科技攻关项目“常压页岩气效益开发技术政策优化研究”（编号：P21087-4）、“渝东南地区浅层页岩气勘探开发关键技术”（编号：P24115）联合资助。

详细信息

作者简介:
周成香（1985—），湖北老河口人，2009年毕业于长江大学石油工程专业，2016年获中国石油大学（北京）石油与天然气工程专业硕士学位，高级工程师，主要从事页岩气勘探开发方面的研究工作。E-mail：278738690@qq.com

中图分类号: TE357.1⁺3
计量
- 文章访问数: 42
- HTML全文浏览量: 4
- PDF下载量: 7
出版历程
- 收稿日期: 2024-03-11
- 修回日期: 2025-01-11
- 网络出版日期: 2025-01-22

The Improvement and Application of Fracturing Technology in the Nanchuan Shale Gas Field

Sinopec Chongqing Shale Gas Co., Ltd., Chongqing, 408400, China

摘要

摘要:
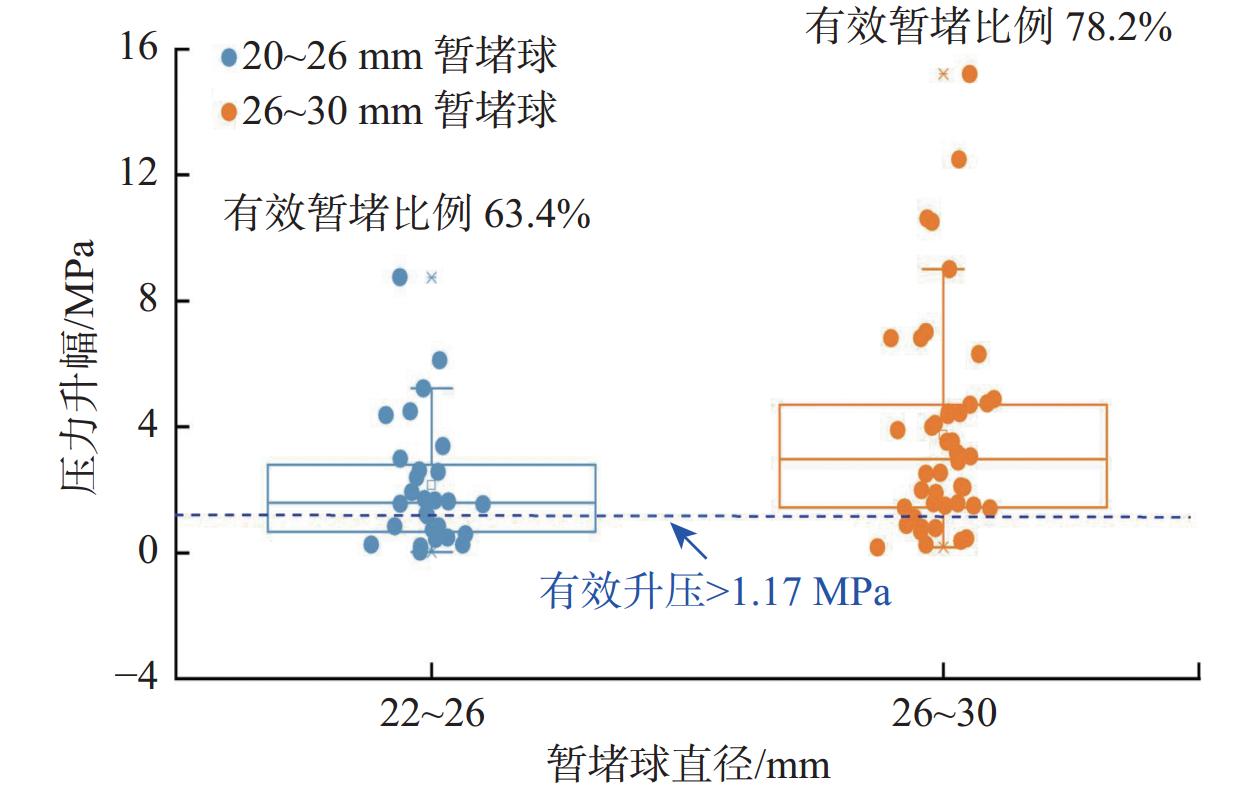
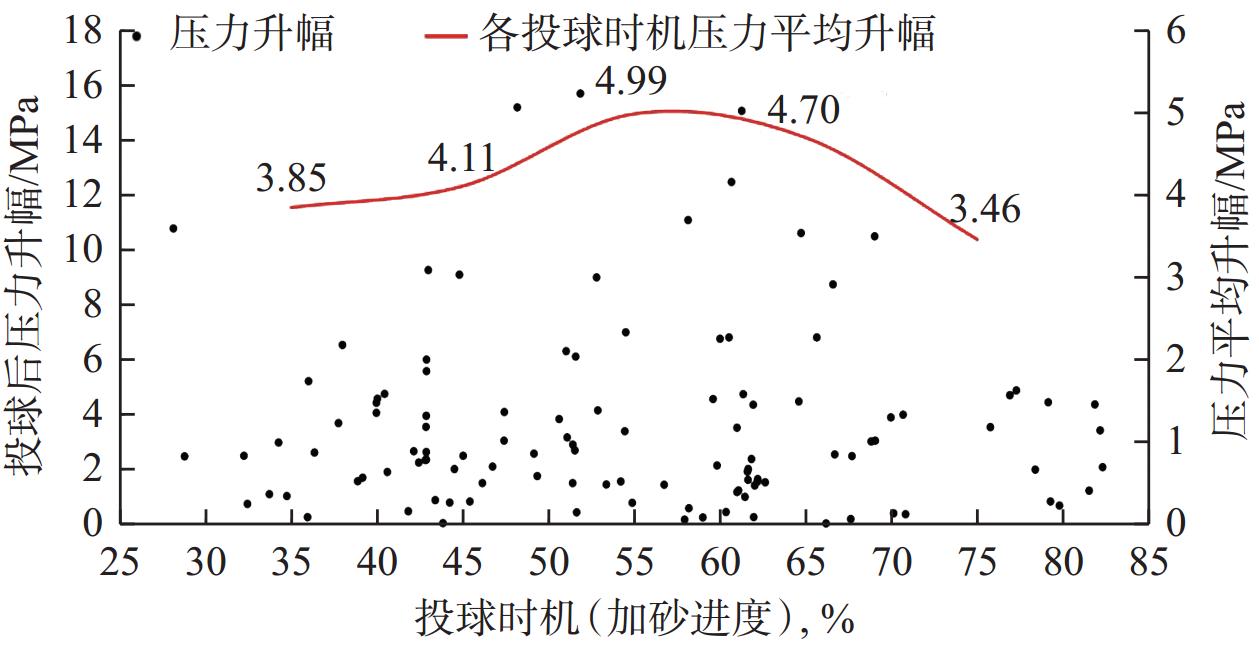
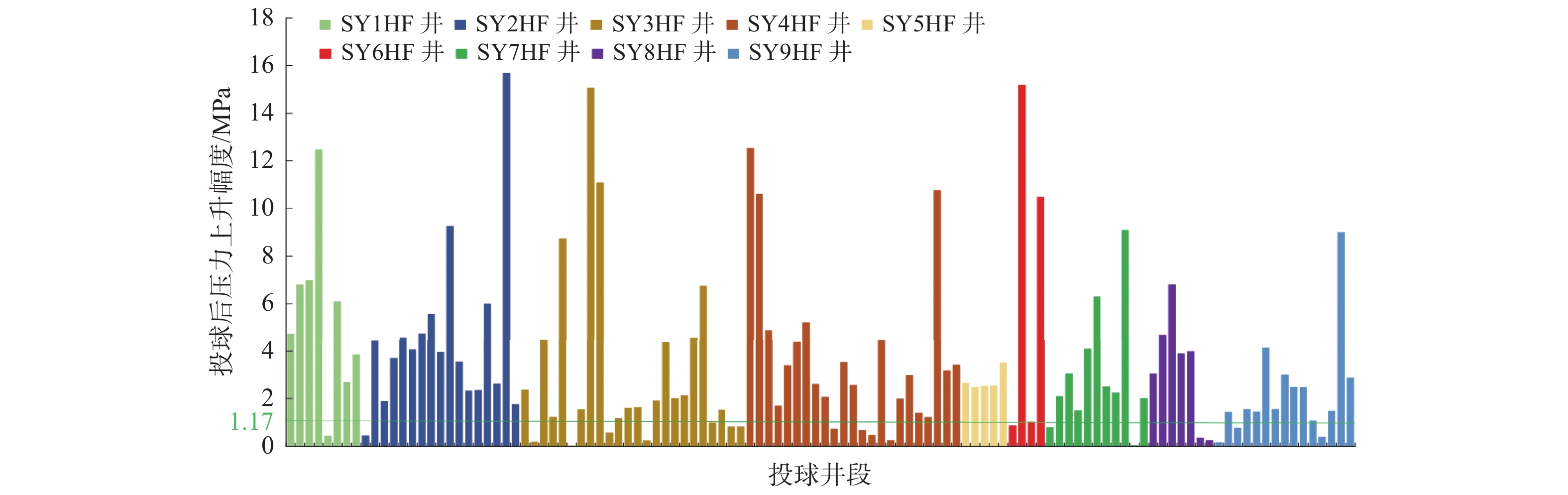
页岩储层具有低孔隙度和特低渗透性特征，必须进行大规模体积压裂改造以形成人工缝网。南川页岩气田开发已经形成相对完善的压裂工艺技术体系，但随着甜点储量规模化生产，亟需深入研究并进一步改进压裂工艺。从南川页岩气田不同井网储量动用、射孔方式、投球暂堵、加砂模式等方面提出了页岩气压裂工艺改进思路和方法，并通过现场应用效果评价了改进工艺的可行性。系统总结了压裂工艺改进措施：鉴于井网关系和开发目标的差异，对不同类型井组的压裂改造区域进行差异化控制；超深穿透射孔方式为深层高应力页岩储层压裂提供了重要的工艺基础，满足了电动压裂设备数量和压力等级的限制；借鉴重复压裂及加密井压裂工艺中的投球暂堵技术，优化了投球数量与时机，抑制了主裂缝过度延伸；精细化压裂，支撑剂体系趋于完善，形成了“初始小粒径远输前缘铺置+中段中粒径主流通道支撑+尾段大粒径缝口收尾”三级连续加砂模式。改进后的压裂工艺现场应用效果显著，在统计的现场投球暂堵中，封堵有效率79.8%，在超深穿透工艺下，为更多加砂和注液提供了压力窗口；提高了加砂强度和小粒径占比，显著提升了裂缝导流能力和支撑效果，压后日产气量从3.30×10⁴ m³提高至8.46×10⁴ m³。研究结果表明，通过综合应用差异化压裂设计、超深穿透射孔技术、优化投球暂堵以及精细化三级加砂模式，可显著提升南川页岩气田的压裂改造效果和经济效益，为南川页岩气田有效开发提供了技术保障。
- 差异化压裂 /
- 超深穿透射孔 /
- 投球暂堵 /
- 加砂模式 /
- 南川页岩气田
Abstract:
Due to the low porosity and extremely low permeability of shale reservoirs, they must undergo large-scale volume fracturing to create an artificial fracture network. The development of the Nanchuan Shale Gas Field has already formed a relatively mature fracturing technology system. However, with the large-scale production of sweet spot reserves, there is an urgent need for in-depth research and further improvement of fracturing technology. Improvement ideas and methods for shale gas technology were proposed for the Nanchuan Shale Gas Field. They involve the utilization of reserves in different well patterns, perforation methods, temporary plugging with ball injection, and sand addition modes. The feasibility of these improvements was evaluated through on-site application effect assessment. Fracturing technology process improvements were summarized systematically. In view of the difference in well pattern and development objectives, the fracturing modification area of different types of well groups was controlled differently. The application of an ultra-deep penetration perforation provided a crucial technological foundation for fracturing in deep and high-stress shale reservoirs, meeting limitations on the number of electric fracturing devices and pressure levels. Repeat fracturing and temporary plugging with ball injection during tight well fracturing resulted in the optimization of the quantity and timing of ball injection to suppress excessive extension of the main fracture. The fracturing process was refined, and the proppant system was improved, leading to a three-stage continuous sand addition mode featuring “long-distance transport placement of initial small particles at front edge + main flow channel support by medium particles in middle section + fracture closure by large particles in tail section.” The improved fracturing technology demonstrated significant on-site application effects. After statistically analyzing the on-site temporary plugging with ball injection, the effective plugging rate was 79.8%. The ultra-deep penetration technology provided a pressure window for more sand addition and fluid injection which increased the sand addition intensity and the proportion of small particles, the fracture conductivity and support effect were significantly enhanced. As a result, the daily production after fracturing increased from 3.30×10⁴ m³ to 8.46×10⁴ m³. It has demonstrated that the integrated application of differentiated fracturing design, ultra-deep penetration perforation technology, optimized temporary plugging with ball injection, and a refined three-stage sand addition mode can significantly enhance the fracturing stimulation effect and economic benefits of the Nanchuan Shale Gas Field. This provides strong technical support for the efficient development of the Nanchuan Shale Gas Field.
- differentiated fracturing /
- ultra-deep penetration perforation /
- temporary plugging with ball injection /
- sand addition model /
- Nanchuan Shale Gas Field

HTML全文

作为非常规能源的页岩气，因资源量巨大得到了广泛关注^[1]，目前主要采用经过大型体积压裂的水平井开发。页岩气储层为泥页岩地层，层理发育，钻井过程中易水化膨胀，发生因井壁失稳引发的卡钻、埋钻等井下故障，严重时可导致井眼报废，造成巨大的经济损失。页岩气水平井井壁失稳问题是制约页岩气安全高效开发的技术瓶颈之一。国内外的专家和学者对此开展了大量的试验和理论研究，并取得了丰富的成果。J. C. Jaeger等人^[2]率先提出了岩石弱面强度失效准则；刘向君等人^[3-5]在此基础上，基于连续介质力学与应力坐标系转换理论，建立了考虑弱面影响的坍塌压力预测模型，分析了弱面稳定性和产状对井壁稳定性的影响；洪国斌等人^[6-7]结合斜井状态下的井周应力模型与弱面破坏准则，建立了大斜度井地层坍塌压力模型，分析了影响井壁稳定性的因素。这些研究仅考虑了弱面对井壁稳定性的影响，未考虑水化作用对井壁稳定性的影响。温航等人^[8]建立了同时考虑层理产状和层理面弱化的坍塌压力模型，分析了影响坍塌压力分布的因素；马天寿、陈平等人^[9-12]结合弱面强度准则，建立了层理性页岩水平井井壁稳定性分析模型，定量分析了层理产状和含水量对水平井坍塌压力的影响。虽然这些研究都同时考虑了层理面和水化作用对泥页岩地层稳定性的影响，但仅考虑了水化作用对岩石本体和弱面力学参数的弱化效应，鲜有考虑水化膨胀应力对井壁稳定性的影响。因此，笔者以弹性力学和岩石力学等理论为基础，既考虑层理面的影响，也考虑水化作用对岩石本体和层理面的强度弱化效应以及水化膨胀应力作用的影响，建立了力化耦合作用下层理性页岩气水平井井壁坍塌压力预测模型，研究了层理性页岩气水平井井壁失稳机理，分析了井眼轨迹、水化应变、层理面产状和钻井时间等因素对坍塌压力的影响规律。

1. 层理页岩水平井坍塌压力预测模型

使用水基钻井液钻进页岩地层时，水基钻井液滤液与页岩地层发生水化反应，导致其强度降低，引发井壁失稳。笔者基于以下假设建立层理性页岩水平井坍塌压力预测模型：

1）不仅考虑水化导致的水化应变对井壁稳定性的影响，还考虑含水量对岩石力学参数的影响；

2）地层岩石为非均质岩石且具有各向同性；

3）岩石除有一组平行的、强度较低的弱面（裂缝）外，其他方向上地层岩石的强度是相同的；

4）岩石变形较小且为线弹性变形。

1.1 考虑水化应变作用的井周应力

水化作用下的井周围岩应力应变（水化应变）平衡方程为：

$\frac{{{\rm{d}}{\sigma _{{rr}}}}}{{{\rm{d}}r}} + \frac{{{\sigma _{rr}} - {\sigma _{\theta \theta }}}}{r} = 0$

(1)

式中：σ_rr和σ_θθ分别为井眼圆柱坐标系下的径向应力和周向应力，Pa；r为半径，m。

井壁上的径向应力等于井内钻井液液柱压力，即内边界条件为σ_rr|_r=R = p_m。距井壁无穷远处的径向应力等于原地应力，即外边界条件为σ_rr|_r→∞ = S。

将内外边界条件代入应力应变平衡方程，最终得到应力应变平衡方程的通解（即径向位移u）为：

$u = Ar + {B / r}$

(2)

$\!{\text{其中}} \quad\qquad A = \dfrac{{\left( {1 - 2\nu } \right)\left( {1 + \nu } \right)}}{E}\dfrac{{\left( {{\sigma _{xx}} + {\sigma _{yy}}} \right)}}{2}\quad\quad$

(3)

$B = \dfrac{{\left( {1 + \nu } \right)}}{{2E}}\left[ {\dfrac{{\left( {{\sigma _{xx}} + {\sigma _{yy}}} \right)}}{2} - {p_{\rm{m}}}} \right]{R^2}$

(4)

式中：σ_xx和σ_yy分别为井眼直角坐标系下x轴和y轴方向的主应力，Pa；u为径向位移，m；p_m为井内有效液柱压力，Pa；E为地层含水量为f_w时的弹性模量，Pa； $\nu$ 为地层含水量为f_w时的泊松比；Pa；R为井眼半径，m。

根据轴对称井筒的平面应变几何方程，可求得水化作用产生的径向和周向水化应变：

$\left\{ \begin{array}{l} {\varepsilon _{rr}} = \dfrac{{{\rm{d}}u}}{{{\rm{d}}r}} = A - \dfrac{B}{{{r^2}}} \\ {\varepsilon _{\theta \theta }} = \dfrac{u}{r} = A + \dfrac{B}{{{r^2}}} \\ \end{array} \right.$

(5)

式中：ε_rr为径向应变；ε_θθ为切向应变。

垂向水化应变需通过相关的试验获取，笔者直接采用C. H. Yew等人^[13]的室内测试结果：

${\varepsilon _{\rm{v}}} = {K_{\rm{1}}}\Delta {f_{\rm{w}}} + {K_{\rm{2}}}\Delta {f_{\rm{w}}}^{\rm{2}}$

(6)

式中：K₁=0.070 8；K₂=11.08；ε_v为垂向水化应变；Δf_w为含水量的增量，Δf_w = f_w–f_wi；f_wi为原始地层含水量。

最终，得到层理性页岩在平面二维空间圆柱坐标系内的水化应力为：

$\left\{ \begin{array}{l} \sigma _{_{rr}}^{{\rm{hy}}} = \dfrac{E}{{(1 - 2\nu )(1 + \nu )}}[(1 - \nu ){\varepsilon _{rr}} + \nu {\varepsilon _{\theta \theta }} - (m + \nu ){\varepsilon _{\rm{v}}}] \\ \sigma _{_{\theta \theta }}^{{\rm{hy}}} = \dfrac{E}{{(1 - 2\nu )(1 + \nu )}}[(1 - \nu ){\varepsilon _{\theta \theta }} + \nu {\varepsilon _{rr}} - (m + \nu ){\varepsilon _{\rm{v}}}] \\ \sigma _{_{zz}}^{{\rm{hy}}} = \dfrac{E}{{(1 - 2\nu )(1 + \nu )}}[\nu {\varepsilon _{rr}} + \nu {\varepsilon _{\theta \theta }} - (1 - \nu + 2m\nu ){\varepsilon _{\rm{v}}}] \end{array} \right.$

(7)

式中： $\sigma _{rr}^{{\rm{hy}}}$ ， $\sigma _{\theta \theta }^{{\rm{hy}}}$ 和 $\sigma _{zz}^{{\rm{hy}}}$ 分别为考虑层理面时井眼圆柱坐标系下的径向应力、周向应力和轴向应力，Pa；m为各向异性比值。

1.2 原地应力产生的井周应力

通过坐标转换得到井周柱坐标系下的主应力和切应力为：

$\left\{\!\!\!\! \begin{array}{l} {\sigma _{rr}} = \dfrac{{{\sigma _{xx}} + {\sigma _{yy}}}}{2}\left(1 - \dfrac{{{R^2}}}{{{r^2}}}\right) + \dfrac{{{\sigma _{xx}} - {\sigma _{yy}}}}{2}\left(1 + \dfrac{{3{R^4}}}{{{r^4}}} - \right. \\ \begin{array}{*{20}{c}} {}&{} \end{array}\left. \dfrac{{4{R^2}}}{{{r^2}}}\right)\cos 2\theta \!+\! {\tau _{xy}}\left(1 \!+\! \dfrac{{3{R^4}}}{{{r^4}}} \!- \! \dfrac{{4{R^2}}}{{{r^2}}}\right)\sin 2\theta \!+\! \dfrac{{{R^2}}}{{{r^2}}}{p_{\rm{m}}} \\ {\sigma _{\theta \theta }} = \dfrac{{{\sigma _{xx}} + {\sigma _{yy}}}}{2}\left(1 + \dfrac{{{R^2}}}{{{r^2}}}\right) - \dfrac{{{\sigma _{xx}} - {\sigma _{yy}}}}{2}\Biggr(1 +\Biggr. \\ \begin{array}{*{20}{c}} {}&{} \end{array} \left. \dfrac{{3{R^4}}}{{{r^4}}}\right) \cos 2\theta - {\tau _{xy}}\left(1 + \dfrac{{3{R^4}}}{{{r^4}}}\right)\sin 2\theta - \dfrac{{{R^2}}}{{{r^2}}}{p_{\rm{m}}} \\ {\sigma _{zz}} = {\sigma _{zz}} - 2v({\sigma _{xx}} - {\sigma _{yy}}{\rm{)}}{\left(\dfrac{R}{r}\right)^2}\cos 2\theta \\ {\tau _{r\theta }} = \left[\dfrac{{({\sigma _{yy}} - {\sigma _{xx}}{\rm{)}}\sin 2\theta }}{2} + {\tau _{xy}}\cos 2\theta \right]\left(1 - \dfrac{{3{R^4}}}{{{r^4}}} +\dfrac{{2{R^2}}}{{{r^2}}}\right)\\ {\tau _{\theta z}} = {\tau _{yz}}\left(1 + \dfrac{{{R^2}}}{{{r^2}}}\right)\cos \theta - {\tau _{xz}}\left(1 + \dfrac{{{R^2}}}{{{r^2}}}\right)\sin \theta \\ {\tau _{rz}} = {\tau _{xz}}\left(1 - \dfrac{{{R^2}}}{{{r^2}}}\right)\cos \theta + {\tau _{yz}}\left(1 - \dfrac{{{R^2}}}{{{r^2}}}\right)\sin \theta \\[-3pt] \end{array} \right.\!\!$

(8)

式中： ${\sigma _{zz}}$ 为井眼圆柱坐标系下的轴向应力，Pa； ${\tau _{r\theta}}$ ， ${\tau _{\theta z}}$ 和 ${\tau _{rz}}$ 为井眼圆柱坐标系下3个平面上的切向应力，Pa； $\theta$ 为沿井眼周向的方位角，（°）。

1.3 井眼总有效应力

井眼总有效应力由2部分组成，一部分是泥页岩水化应变产生的水化应力，另一部分是由原地应力产生的应力，计算公式为：

$\left\{ \begin{array}{l} \sigma _{rr}^{\rm{t}} = {\sigma _{rr}} + \sigma _{rr}^{{\rm{hy}}} \\ \sigma _{\theta \theta }^{\rm{t}} = {\sigma _{\theta \theta }} + \sigma _{\theta \theta }^{{\rm{hy}}} \\ \sigma _{_{zz}}^t = {\sigma _{zz}} + \sigma _{zz}^{{\rm{hy}}} \\ \tau _{_{r\theta }}^{\rm{t}} = {\tau _{r\theta }} \\ \tau _{_{qz}}^{\rm{t}} = {\tau _{\theta z}} \\ \tau _{_{rz}}^{\rm{t}} = {\tau _{rz}} \\ \end{array} \right.$

(9)

当r=R时，即可得到井壁上的总有效应力：

$\left\{ \begin{array}{l} \sigma _{_{rr}}^{\rm{t}}\! = \! \dfrac{E}{{(1 - 2v)(1 + v)}}[(1 - v){\varepsilon _{rr}} + v{\varepsilon _{\theta \theta }} - (m + v){\varepsilon _{\rm{v}}}] +\\ \qquad\; {p_{\rm{m}}} - \eta {p_{\rm{p}}} \\ \sigma _{_{\theta \theta }}^{\rm{t}} \! = \! \dfrac{E}{{(1 - 2v)(1 + v)}}[(1 - v){\varepsilon _{\theta \theta }} + \nu {\varepsilon _{rr}} - (m + v){\varepsilon _{\rm{v}}}] + \\\\ \begin{array}{*{20}{c}} {}&{} \end{array} \; {\sigma _{xx}}(1 - 2\cos \theta ) + {\sigma _{yy}}(1 + 2\cos 2\theta )- \\ \qquad\; 4{\tau _{xy}} \sin 2\theta - {p_{\rm{m}}} - \eta {p_{\rm{p}}} \\ \sigma _{{}_{zz}}^{\rm{t}} \! = \! \dfrac{E}{{(1 - 2v)(1 + v)}}[v{\varepsilon _{rr}} + v{\varepsilon _{\theta \theta }} - (1 - v + 2mv){\varepsilon _{\rm{v}}}] + \\\\ \begin{array}{*{20}{c}} {}&{} \end{array} \, {\sigma _{zz}} - v[2({\sigma _{xx}} - {\tau _{xy}})\cos 2\theta + 4{\tau _{xy}}\sin 2\theta ] - \eta {p_{\rm{p}}} \\ \tau _{_{\theta z}}^{\rm{t}} = 2{\tau _{yz}}\cos \theta - 2{\tau _{xz}}\sin \theta \\ \tau _{_{r\theta }}^{\rm{t}} = 0 \\ \tau _{_{rz}}^{\rm{t}} = 0 \\ \end{array} \right.$

(10)

式中： $\sigma _{rr}^{\rm{t}}$ ， $\sigma _{\theta \theta }^{\rm{t}}$ ， $\sigma _{zz}^{\rm{t}}$ ， $\tau _{r\theta }^{\rm{t}}$ ， $\tau _{\theta z}^{\rm{t}}$ 和 $\tau _{rz}^{\rm{t}}$ 为考虑水化应力作用后的井眼总有效应力分量，Pa；η为有效应力系数；p_p为地层孔隙压力，Pa。

由式（8）可知， $\sigma _{rr}^{\rm{t}}$ 是其中一个主应力，即 ${\sigma _{\rm{r}}}=\sigma _{rr}^{\rm{t}}$ 。另外2个主应力的计算公式为：

$\left\{ \begin{array}{l} {\sigma _{\rm{a}}} = \dfrac{{\sigma _{zz}^{\rm{t}} + \sigma _{\theta \theta }^{\rm{t}}}}{2} + \sqrt {{{\left(\dfrac{{\sigma _{zz}^{\rm{t}} - \sigma _{\theta \theta }^{\rm{t}}}}{2}\right)}^2} + {{(\tau _{\theta z}^{\rm{t}})}^2}} \\ {\sigma _{\rm{b}}} = \dfrac{{\sigma _{zz}^{\rm{t}} + \sigma _{\theta \theta }^{\rm{t}}}}{2} - \sqrt {{{\left(\dfrac{{\sigma _{zz}^{\rm{t}} - \sigma _{\theta \theta }^{\rm{t}}}}{2}\right)}^2} + {{(\tau _{\theta z}^{\rm{t}})}^2}} \\ \end{array} \right.$

(11)

则最大和最小主应力为：

$\left\{ \begin{array}{l} {\sigma _{\rm{1}}} = \max ({\sigma _{\rm{a}}},{\sigma _{\rm{b}}},{\sigma _{\rm{r}}}) \\ {\sigma _{\rm{3}}} = \min ({\sigma _{\rm{a}}},{\sigma _{\rm{b}}},{\sigma _{\rm{r}}}) \\ \end{array} \right.$

(12)

式中： ${\sigma _{\rm{a}}}$ ， ${\sigma _{\rm{b}}}$ 和 ${\sigma _{\rm{r}}}$ 为井壁上的主应力，Pa； ${\sigma _1}$ 和 ${\sigma _3}$ 分别为最大、最小主应力，Pa。

通过以上模型可以求得页岩气水平井井壁上的最大主应力和最小主应力，再结合Mohr-Coulomb弱面强度准则即可求得坍塌压力。

1.4 坍塌压力的确定

层理性地层具有一组近似平行的层理面，层理面的强度低于岩石本体强度，又被称之为弱面。J. C. Jaeger在1960年提出了单一弱面强度理论，该理论是对Mohr-Coulomb破坏准则的推广，可描述具有一条或一组平行弱面的各向同性岩体的剪切破坏（见图1）。笔者以单一弱面强度理论作为井壁失稳的判定依据。

图 1 单一弱面剪切失效分析示意

Figure 1. Analysis of shear failure on single weak plane

下载: 全尺寸图片幻灯片

${\sigma _{\rm{1}}} - {\sigma _{\rm{3}}} = \frac{{2\left( {{C_{\rm{w}}} + {\sigma _{\rm{3}}}\tan {\varphi _{\rm{w}}}} \right)}}{{\left( {1 - \tan {\varphi _{\rm{w}}}\cot \beta } \right)\sin 2\beta }}$

(13)

$\beta = {\rm{arc}}\cos \frac{{{{n}} \cdot {{N}}}}{{\left| {{n}} \right| \cdot \left| {{N}} \right|}}$

(14)

${{n}} = \sin {i_{\rm{w}}}\cos {\alpha _{\rm{w}}}{{i}} + \sin {i_{\rm{w}} }\sin {\alpha _{\rm{w}} }{{j}} + \cos {\alpha _{\rm{w}} }{{k}}$

(15)

${{N}} = \left\{ \begin{array}{l} \sin \gamma {{j}} + \cos \gamma {{k}} \\ - \cos \gamma {{j}} + \sin \gamma {{k}} \\ \end{array} \right.\begin{array}{*{20}{c}} {}&\begin{array}{l} {\sigma _{_{zz}}^{\rm{t}} \geqslant \sigma _{\theta \theta }^{\rm{t}}} \\ {\sigma _{_{zz}}^{\rm{t}} < \sigma _{\theta \theta }^{\rm{t}}} \\ \end{array} \end{array}$

(16)

$\gamma = \left\{ \begin{array}{l} \dfrac{{\text{π}}}{4} \\ 0.5\arctan \left| {\dfrac{{2\tau _{_{\theta z}}^{\rm{t}}}}{{\sigma _{\theta \theta }^{\rm{t}} - \sigma _{_{zz}}^{\rm{t}}}}} \right| \\ \end{array} \right.\begin{array}{*{20}{c}} {}&\begin{array}{l} {\sigma _{_{zz}}^{\rm{t}} = \sigma _{_{\theta \theta }}^{\rm{t}}} \\ {\sigma _{_{zz}}^{\rm{t}} \ne \sigma _{_{\theta \theta }}^{\rm{t}}} \\ \end{array} \end{array}$

(17)

弱面破坏时β的下限（β₁）和上限（β₂）分别为：

$\left\{ \begin{array}{l} {\beta _{\rm{1}}} = 0.5{\varphi _{\rm{w}}} + 0.5\arcsin \left[{\dfrac{{({\sigma _{\rm{1}}} + {\sigma _{\rm{3}}} + 2{C_{\rm{w}}}\cot {\varphi _{\rm{w}}})\sin {\varphi _{\rm{w}}}}}{{{\sigma _{\rm{1}}} - {\sigma _{\rm{3}}}}}} \right] \\ {\beta _{\rm{2}}} = \dfrac{{\text{π}}}{2} + {\varphi _{\rm{w}}} - {\beta _{\rm{1}}} \\ \end{array} \right.$

(18)

β₁和β₂分别为弱面破坏时β的下限和上限。由摩尔应力圆可知：当β₁≤β≤β₂时，弱面破坏造成井壁失稳；当0<β<β₁或β₂<β<π/2时，弱面稳定，此时岩石本体破坏造成井壁失稳。判定岩石本体破坏的依据为：

${\sigma _{\rm{1}}} - {\sigma _{\rm{3}}} = \frac{{2\cos \varphi }}{{1 - \sin \varphi }} + {\sigma _{\rm{3}}}\frac{{1 + \sin \varphi }}{{1 - \sin \varphi }}$

(19)

式中：β为弱面法线与最大主应力夹角，（°）；C_w为层理面黏聚力，MPa； ${\varphi _{\rm{w}}}$ 为层理面内摩擦角，（°）；α_w为层理面倾向，（°）；i_w为层理面倾角，（°）。

黄荣樽等人^[14]利用泥页岩岩心不同含水量下的力学参数试验数据求得了泥页岩力学特性参数随含水量的变化规律：

$\left\{ \begin{array}{l} E = {E_{\rm{0}}}{{\rm{e}}^{ - 11\sqrt {{f_{\rm{w}}} - {f_{\rm{w}}}_{\rm{i}}} }} \\ \nu = {\nu _{\rm{0}}} + 1.3{f_{\rm{w}}} \\ C = {C_{\rm{0}}} - 58.8\left( {{f_{\rm{w}}} - {f_{\rm{w}}}_{\rm{i}}} \right) \\ \varphi = {\varphi _{\rm{0}}} - 187.5\left( {{f_{\rm{w}}} - {f_{\rm{w}}}_{\rm{i}}} \right) \\ \end{array} \right.$

(20)

式中：E₀为初始弹性模量，Pa；v₀为初始泊松比；C₀为原始黏聚力，Pa； ${\varphi _0}$ 为原始内摩擦角，（°）； $\nu$ 为地层含水量为 ${f_{\rm{w}}}$ 时的泊松比；C为地层含水量为 ${f_{\rm{w}}}$ 时的黏聚力，Pa； $\varphi$ 为地层含水量为 ${f_{\rm{w}}}$ 时的内摩擦角，（°）。

2. 坍塌压力模型求解

笔者利用Matlab软件编制了利用迭代法求取坍塌压力（用坍塌压力当量密度表示，下文简称为当量密度）的程序，用于预测力化耦合条件下考虑层理面影响的页岩气水平井坍塌压力，计算程序的求解流程如图2所示。

图 2 坍塌压力求解流程

Figure 2. Solution flow of collapse pressure

下载: 全尺寸图片幻灯片

图3为考虑与不考虑水化应变时坍塌压力当量密度计算结果。由图3可知：在当前计算条件下，无论是否考虑水化应变，沿最小水平主应力方向（方位角90°）钻进，且井斜角相对较大（>60°）时，井壁最易失稳（见图3（a）和图3（b））；考虑水化应变时的当量密度普遍大于不考虑水化应变时的当量密度（见图3（c）和图3（d）），其最大差值大于1.72 kg/L，当方位角相对较大（>180°）时，考虑与不考虑水化应变的当量密度差相对较大。

图 3 考虑与不考虑水化应变时的坍塌压力当量密度（i_w=45°，α_w=45°）

Figure 3. Equivalent density of collapse pressure with and without considering hydration strain (i_w=45°，α_w=45°)

下载: 全尺寸图片幻灯片

综上所述，使用水基钻井液钻进层理性泥页岩地层时，水化应变对坍塌压力的影响相对较大，在设计钻井液密度时，不仅要考虑水化作用对岩石力学特性参数的影响，还需要同时考虑水化应变（或水化应力）对坍塌压力的影响。

3. 力化耦合下层理性页岩井壁失稳影响因素分析

笔者采用以下基础参数系统分析地应力机制、井斜角、方位角、弱面产状、地层岩石力学参数和水化特征等影响力化耦合作用下层理性页岩地层坍塌压力的规律：井眼半径为0.108 m，井深为2 420.0 m，最大水平主应力为76.30 MPa，最大水平主应力方位角为0°，最小水平主应力为55.14 MPa，垂向主应力为61.96 MPa，地层孔隙压力为21.48 MPa，初始泊松比为0.2，有效应力系数为0.8，原始地层含水量为0.034，井壁含水量为0.054，岩石本体原始黏聚力为16.61 MPa，岩石本体原始内摩擦角为32.76°，层理面黏聚力为5.28 MPa，层理面内摩擦角为20.81°，层理面倾向为135°，层理面倾角为0°，地层各向异性比值为0.71，井斜角为0°～90°，井眼方位角为0°～360°。

图4为不同条件下的坍塌压力当量密度计算结果。由图4可知：在无弱面和水化作用时，定向井或水平井沿最大水平主应力方向钻进最有利于井壁稳定（见图4（a））；仅考虑弱面影响时，井斜角越小越有利于井壁稳定（见图4（b））；当仅考虑水化作用时，坍塌压力当量密度随井斜角和方位角的变化趋势与不考虑弱面和水化作用时几乎相同（见图4（c））；与原始条件相比，当存在弱面或水化作用时，会使坍塌压力当量密度大幅度升高，井壁易失稳；当同时考虑弱面和水化作用时，沿最小水平主应力方向钻进，且井斜角在40°左右时，井壁最易失稳（见图4（d））。

图 4 不同条件下的坍塌压力当量密度

Figure 4. Equivalent density of collapse pressure under different conditions

下载: 全尺寸图片幻灯片

3.1 地应力机制对坍塌压力的影响

在保持3个主地应力不变的情况下，采用3种不同的地应力机制（σ_H>σ_V>σ_h，σ_H>σ_h>σ_V和σ_V>σ_H>σ_h），分析了坍塌压力当量密度随井斜角和方位角的变化规律，结果如图5所示。由图5可知：无论在哪种地应力机制下，直井段的井壁最稳定，当井斜角在45°附近时，井壁最易失稳；在正断层地应力机制下（σ_V>σ_H>σ_h），井壁最稳定，在走滑断层地应力机制下（σ_H>σ_V>σ_h）和逆断层地应力机制下（σ_H>σ_h>σ_V）井壁最易失稳；在走滑断层地应力机制下和逆断层地应力机制下，当方位角为270°～90°时，井壁最易失稳，在正断层地应力机制下，方位角为60°～240°时，井壁最易失稳。

图 5 不同地应力机制下坍塌压力当量密度随井斜角和方位角的变化规律

Figure 5. Variation of equivalent density of collapse pressure with deviation angle and azimuth under different crustal stress mechanisms

下载: 全尺寸图片幻灯片

3.2 层理面产状对坍塌压力的影响

分析不同井斜角和方位角下，坍塌压力当量密度随弱面倾角和倾向的变化规律，结果见图6。由图6可知：无论井斜角和方位角如何变化，当弱面倾角和倾向与井斜角和方位角一致时，最有利于井壁稳定；当i=45°，且弱面倾角在90°附近和弱面倾向在45°、135°、225°和315°附近时井壁最易失稳；当i=90°，且弱面倾向与井眼垂直时，井壁最易失稳。

图 6 不同井斜角和方位角下层理面产状对坍塌压力当量密度的影响

Figure 6. Effect of bedding occurrence on equivalent density of collapse pressure under different deviation angle and azimuth

下载: 全尺寸图片幻灯片

3.3 含水量对坍塌压力的影响

分析了不同含水量下坍塌压力当量密度随井斜角和方位角的变化规律，结果见图7。由图7可知：井壁最稳定区域和易失稳区域几乎不随含水量增大而变化，原因可能是：在给定计算条件下，含水量增大对岩石力学参数的弱化效应大于水化膨胀应力的变化，从而导致井壁最稳定区域和最易失稳区域几乎不随含水量变化而变化；不同井斜角和方位角所对应的坍塌压力当量密度随含水量增大而升高，原因可能是：水化应力和水化作用对岩石力学参数的弱化程度均随含水量增大而增强，最终导致坍塌压力当量密度随含水量增大而升高。

图 7 不同含水量下坍塌压力当量密度随井斜角和方位角的变化规律

Figure 7. Variation of equivalent density of collapse pressure with deviation angel and azimuth under different water cut

下载: 全尺寸图片幻灯片

3.4 水化时间对坍塌压力的影响

图8为坍塌压力当量密度随水化时间和径向距离变化的分析结果。由图8可知：近井壁处的坍塌压力当量密度随水化时间增长而升高；当水化时间相同时，坍塌压力当量密度随离井壁径向距离增大而降低；井壁易失稳区域随着水化时间增长而增大；井壁上的坍塌压力当量密度不随水化时间变化而变化，因为井壁上的含水量不随水化时间变化而变化。

图 8 坍塌压力当量密度随水化时间和径向距离的变化规律（i=90°，α=0°）

Figure 8. Variation of equivalent density of collapse pressure with hydration time and radial distance（i=90°，α=0°）

下载: 全尺寸图片幻灯片

4. 结论及建议

1）考虑层理面效应后，坍塌压力当量密度大幅升高；沿层理面方位钻进时，井壁最稳定。

2）无论在哪种地应力机制下，直井段（沿层里面钻井）的井壁最稳定，当井斜角在45°附近时，井壁最易失稳。

3）含水量变化不会对最稳定区域或易失稳区域产生影响；在相同条件下，坍塌压力当量密度随含水量增大而升高。

4）近井壁地层的坍塌压力当量密度随水化时间增长而升高；当水化时间相同时，坍塌压力当量密度随距井壁径向距离增大而降低。

5）由于使用水基钻井液钻进层理性页岩地层时，水化应变对坍塌压力当量密度的影响较大，因此，建议在设计钻井液密度时，既要考虑水化作用对岩石力学参数的影响，同时也要考虑水化膨胀应力的影响。在钻井过程中要严格控制钻井液的滤失量，提高钻井液的抑制性，以降低地层含水量，减小水化应变，确保井壁稳定。

图 1 不同改造强度下邻井套压升幅

Figure 1. Increase in casing pressure in adjacent well under different stimulation intensities

下载: 全尺寸图片幻灯片

图 2 缝网与生产压力模拟（15年）结果

Figure 2. Fracture networks and production pressure (15 years) simulation results

下载: 全尺寸图片幻灯片

图 3 加密井开发归一化生产曲线

Figure 3. Normalized production curve for development of encrypted wells

下载: 全尺寸图片幻灯片

图 4 不同加砂强度下的缝网模拟结果

Figure 4. Fracture network simulation results under different sand addition intensities

下载: 全尺寸图片幻灯片

图 5 缝网属性与改造规模的关系

Figure 5. Relationship between fracture network properties and stimulation scale

下载: 全尺寸图片幻灯片

图 6 DFN模型切片和微地震事件分布

Figure 6. DNF model slice and microseismic event distribution

下载: 全尺寸图片幻灯片

图 7 破裂压力与射孔深度的关系曲线

Figure 7. Variation of fracturing pressure with perforation depth

下载: 全尺寸图片幻灯片

图 8 射孔弹穿深试验井段压裂施工曲线

Figure 8. Fracturing construction curve in perforation penetration depth test

下载: 全尺寸图片幻灯片

图 9 不同球径暂堵球的升压情况对比

Figure 9. Comparison of initiation pressures for temporary plugging balls with different diameters

下载: 全尺寸图片幻灯片

图 10 不同球孔比暂堵球的升压情况对比

Figure 10. Comparison of initiation pressure for temporary plugging balls with different ball-to-hole ratios

下载: 全尺寸图片幻灯片

图 11 不同投球时机下暂堵球的升压情况对比

Figure 11. Comparison of initiation pressures for temporary plugging balls at different ball injection timings

下载: 全尺寸图片幻灯片

图 12 井段微地震事件分布

Figure 12. Distribution of microseismic events in the well segment

下载: 全尺寸图片幻灯片

图 13 南川页岩气田平均加砂强度变化

Figure 13. Variation of average sand addition intensity in the Nanchuan Shale Gas Field

下载: 全尺寸图片幻灯片

图 14 部分应用井投球暂堵起裂压力情况统计结果

Figure 14. Statistical analysis of initiation pressure for some application wells during temporary plugging with ball injection

下载: 全尺寸图片幻灯片

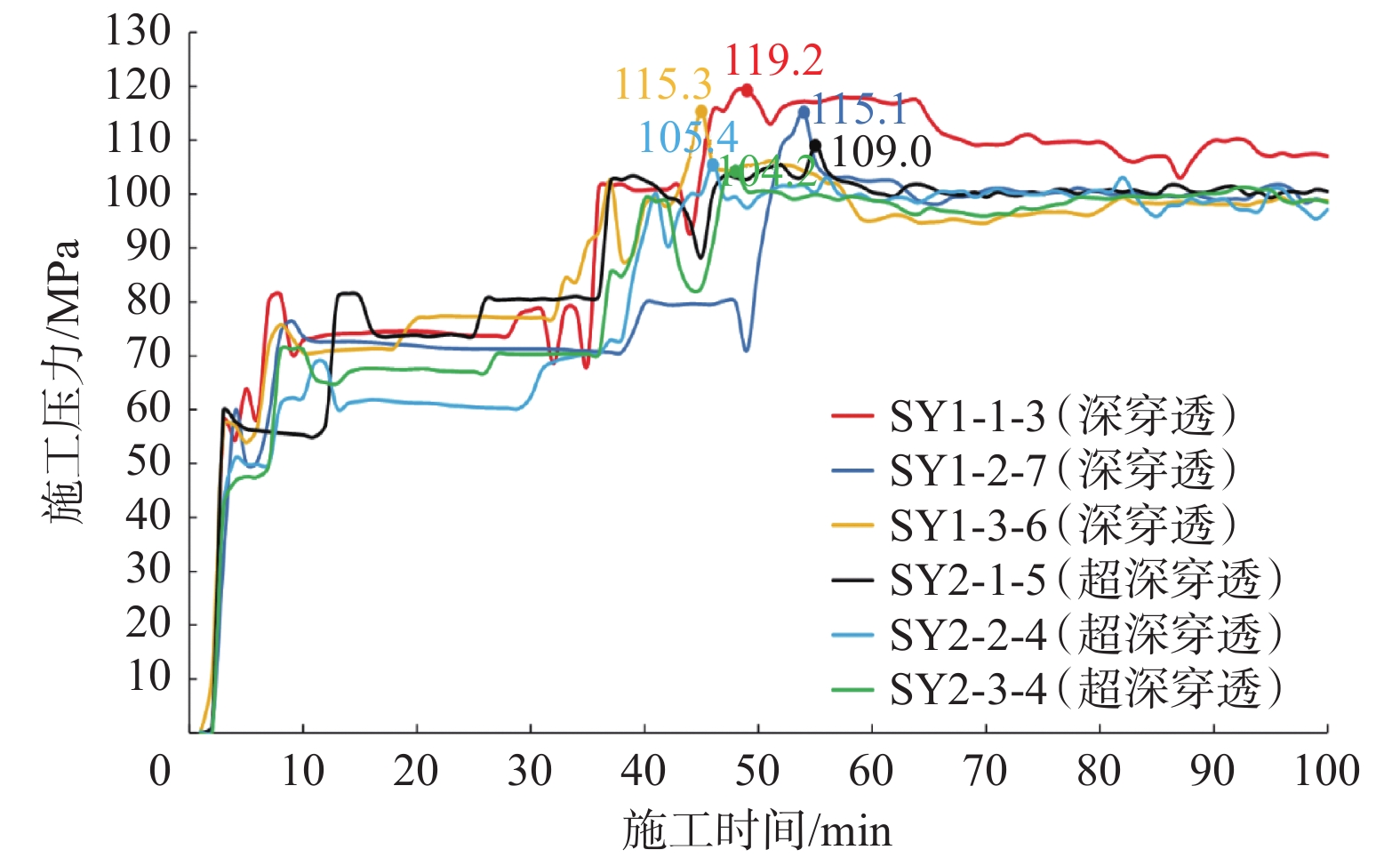
表 1 层段矿物组成与力学参数

Table 1 Mineral composition and mechanical parameters of the rock layers

井段	埋深/m	层位	小层	矿物含量，%					弹性模量/MPa	泊松比	水平主应力/MPa
井段	埋深/m	层位	小层	硅质	钙质	泥质	石英	碳酸盐	弹性模量/MPa	泊松比	最小	最大
SY1−1−3	4 314	五峰组—龙马溪组	③	55.5	2.0	22.7	35.44	10.74	58.30	0.150	90.19	102.82
SY1−2−7	4 407	五峰组—龙马溪组	③	48.1	8.4	32.4	39.36	7.46	54.22	0.158	89.93	102.16
SY1−3−6	4 464	五峰组—龙马溪组	③	48.7	14.3	24.8	45.30	6.90	55.06	0.160	91.85	103.45
SY2−1−5	4 392	五峰组—龙马溪组	③	44.0	6.5	34.9	50.01	7.83	56.78	0.165	90.52	101.96
SY2−2−4	4 412	五峰组—龙马溪组	③	46.8	7.7	30.1	50.41	5.10	50.32	0.165	90.33	102.52
SY2−3−4	4 388	五峰组—龙马溪组	③	46.9	11.7	31.0	44.30	16.60	54.36	0.160	89.87	101.19

下载: 导出CSV

表 2 微地震监测数据

Table 2 Microseismic monitoring data

井号	压裂段	类型	微地震事件数量	M-SRV/ 10⁴ m³	E-SRV/ 10⁴ m³
SY2HF	1～6，8～10，16，18～22	非暂堵	112	297	168
SY2HF	7，11～15，17，23～32	暂堵	107	313	174
SY3HF	1～4	非暂堵	75	300	190
SY3HF	5～27	暂堵	101	422	280
SY4HF	1～3，5，7，11～15	非暂堵	139	377	203
SY4HF	4，6，8～10，16～22	暂堵	154	480	228
SY7HF	1～4，9～14，20，21，24～26	非暂堵	85	261	127
SY7HF	5～8，15～19，22，23	暂堵	94	296	143

下载: 导出CSV

表 3 部分应用井的压裂施工参数

Table 3 Fracturing parameters of some application wells

工艺	井号	类型	储层埋深/ m	平均段长/ m	射孔类型	暂堵类型	加砂强度/ （m³∙m⁻¹）	注液强度/ （m³∙m⁻¹）	小粒径占比，%	稳定测试产量/ （10⁴m³∙d⁻¹）
常规	SY14−8HF	加密井	3 352	99	常规射孔	无暂堵	1.31	27.24	38	3.30
投球暂堵	SY14−6HF	加密井	3 363	105	常规射孔	投球暂堵	1.23	25.91	33	6.23
投球暂堵	SY14−7HF	加密井	3 443	109	常规射孔	投球暂堵	1.17	23.94	33	6.30
深穿透+中等加砂+ 小粒径高占比	SY35−1HF	加密井	4 202	84	深穿透	无暂堵	2.05	20.14	76	8.20
	SY35−2HF	加密井	4 071	79	深穿透	无暂堵	2.02	20.42	82	6.71
	SY35−3HF	加密井	3 973	85	深穿透	无暂堵	2.04	20.44	74	8.46
	SY35−4HF	加密井	3 834	87	深穿透	无暂堵	2.08	21.59	79	8.40
超深穿透+中等加砂+ 小粒径高占比	SY36−1HF	加密井	4 294	86	超深穿透	无暂堵	2.19	26.04	79	7.80
	SY36−2HF	加密井	4 185	72	超深穿透	无暂堵	2.22	28.40	89	7.88
	SY36−4HF	加密井	4 093	85	超深穿透	无暂堵	2.20	22.31	75	7.64
投球暂堵+高液强砂	SY4−1HF	扩边井	2 031	92	常规射孔	投球暂堵	4.51	33.12	15	9.76
	SY4−3HF	扩边井	1 914	114	常规射孔	投球暂堵	4.02	35.06	34	9.30
	SY4−4HF	扩边井	1 869	66	常规射孔	投球暂堵	3.93	31.91	35	8.60

下载: 导出CSV

参考文献(31)

[1]	聂海宽,党伟,张珂,等. 中国页岩气研究与发展20年:回顾与展望[J]. 天然气工业,2024,44(3):20–52. NIE Haikuan, DANG Wei, ZHANG Ke, et al. Two decades of shale gas research & development in China: review and prospects[J]. Natural Gas Industry, 2024, 44(3): 20–52.
[2]	邹才能,赵群,丛连铸,等. 中国页岩气开发进展、潜力及前景[J]. 天然气工业,2021,41(1):1–14. ZOU Caineng, ZHAO Qun, CONG Lianzhu, et al. Development progress, potential and prospect of shale gas in China[J]. Natural Gas Industry, 2021, 41(1): 1–14.
[3]	王光付,李凤霞,王海波,等. 四川盆地不同类型页岩气压裂难点和对策[J]. 石油与天然气地质,2023,44(6):1378–1392. doi: 10.11743/ogg20230604 WANG Guangfu, LI Fengxia, WANG Haibo, et al. Difficulties and countermeasures for fracturing of various shale gas reservoirs in the Sichuan Basin[J]. Oil & Gas Geology, 2023, 44(6): 1378–1392. doi: 10.11743/ogg20230604
[4]	易良平,杨长鑫,杨兆中,等. 天然裂缝带对深层页岩压裂裂缝扩展的影响规律[J]. 天然气工业,2022,42(10):84–97. doi: 10.3787/j.issn.1000-0976.2022.10.008 YI Liangping, YANG Changxin, YANG Zhaozhong, et al. Influence of natural fracture zones on the propagation of hydraulic fractures in deep shale[J]. Natural Gas Industry, 2022, 42(10): 84–97. doi: 10.3787/j.issn.1000-0976.2022.10.008
[5]	何希鹏,何贵松,高玉巧,等. 常压页岩气勘探开发关键技术进展及攻关方向[J]. 天然气工业,2023,43(6):1–14. HE Xipeng, HE Guisong, GAO Yuqiao, et al. Progress in and research direction of key technologies for normal-pressure shale gas exploration and development[J]. Natural Gas Industry, 2023, 43(6): 1–14.
[6]	姚红生,王伟,何希鹏,等. 南川复杂构造带常压页岩气地质工程一体化开发实践[J]. 油气藏评价与开发,2023,13(5):537–547. YAO Hongsheng, WANG Wei, HE Xipeng, et al. Development practices of geology-engineering integration in complex structural area of Nanchuan normal pressure shale gas field[J]. Petroleum Reservoir Evaluation and Development, 2023, 13(5): 537–547.
[7]	姚红生,房启龙,袁明进,等. 渝东南常压页岩气工程工艺技术进展及下一步攻关方向[J]. 石油实验地质,2023,45(6):1132–1142. doi: 10.11781/sysydz2023061132 YAO Hongsheng, FANG Qilong, YUAN Mingjin, et al. Progress of normal-pressure shale gas engineering technology in southeast Chongqing and the research direction of next steps[J]. Petroleum Geology and Experiment, 2023, 45(6): 1132–1142. doi: 10.11781/sysydz2023061132
[8]	倪锋,朱峰,孟庆利. 渝东南地区南川区块膝折构造模式解析[J]. 油气藏评价与开发,2024,14(3):373–381. NI Feng, ZHU Feng, MENG Qingli. Analysis of knee fold structure model in Nanchuan Block of southeastern Chongqing[J]. Petroleum Reservoir Evaluation and Development, 2024, 14(3): 373–381.
[9]	张莉娜,任建华,胡春锋. 常压页岩气立体开发特征及缝网干扰规律研究[J]. 石油钻探技术,2023,51(5):149–155. ZHANG Lina, REN Jianhua, HU Chunfeng. Three-dimensional development characteristics and fracture network interference of atmospheric shale gas reservoir[J]. Petroleum Drilling Techniques, 2023, 51(5): 149–155.
[10]	周博成,熊炜,赖建林,等. 武隆区块常压页岩气藏低成本压裂技术[J]. 石油钻探技术,2022,50(3):80–85. ZHOU Bocheng, XIONG Wei, LAI Jianlin, et al. Low-cost fracturing technology in normal-pressure shale gas reservoirs in Wulong Block[J]. Petroleum Drilling Techniques, 2022, 50(3): 80–85.
[11]	李龙,陈显举,彭安钰,等. 贵州正安地区常压页岩气压裂关键技术[J]. 钻探工程,2022,49(5):189–193. LI Long, CHEN Xianju, PENG Anyu, et al. Key technologies for hydraulic fracturing of normal pressure shale gas in the Zheng'an Area of Guizhou[J]. Drilling Engineering, 2022, 49(5): 189–193.
[12]	刘洪,廖如刚,李小斌,等. 页岩气 “井工厂” 不同压裂模式下裂缝复杂程度研究[J]. 天然气工业,2018,38(12):70–76. LIU Hong, LIAO Rugang, LI Xiaobin, et al. A comparative analysis on the fracture complexity in different fracking patterns of shale gas “well factory”[J]. Natural Gas Industry, 2018, 38(12): 70–76.
[13]	何希鹏,张培先,任建华,等. 渝东南南川地区东胜构造带常压页岩气勘探开发实践[J]. 石油实验地质,2023,45(6):1057–1066. HE Xipeng, ZHANG Peixian, REN Jianhua, et al. Exploration and development practice of normal pressure shale gas in Dongsheng Structural Belt, Nanchuan Area, southeast Chongqing[J]. Petroleum Geology and Experiment, 2023, 45(6): 1057–1066.
[14]	蒋恕,李园平,杜凤双,等. 提高页岩气藏压裂井射孔簇产气率的技术进展[J]. 油气藏评价与开发,2023,13(1):9–22. JIANG Shu, LI Yuanping, DU Fengshuang, et al. Recent advancement for improving gas production rate from perforated clusters in fractured shale gas reservoir[J]. Petroleum Reservoir Evaluation and Development, 2023, 13(1): 9–22.
[15]	郭建春,路千里,刘壮,等. “多尺度高密度”压裂技术理念与关键技术:以川西地区致密砂岩气为例[J]. 天然气工业,2023,43(2):67–76. GUO Jianchun, LU Qianli, LIU Zhuang, et al. Concept and key technology of “multi-scale high-density” fracturing technology: a case study of tight sandstone gas reservoirs in the western Sichuan Basin[J]. Natural Gas Industry, 2023, 43(2): 67–76.
[16]	刘善勇,尹彪,楼一珊,等. 粗糙裂缝内支撑剂运移与展布规律数值模拟[J]. 石油钻探技术,2024,52(4):104–109. LIU Shanyong, YIN Biao, LOU Yishan, et al. Numerical simulation of migration and placement law of proppants in rough fractures[J]. Petroleum Drilling Techniques, 2024, 52(4): 104–109.
[17]	唐堂,郭建春,翁定为,等. 基于PIV/PTV的平板裂缝支撑剂输送试验研究[J]. 石油钻探技术,2023,51(5):121–129. TANG Tang, GUO Jianchun, WENG Dingwei, et al. Experimental study of proppant transport in flat fracture based on PIV/PTV[J]. Petroleum Drilling Techniques, 2023, 51(5): 121–129.
[18]	刘建坤,蒋廷学,卞晓冰,等. 常压页岩气低成本高效压裂技术对策[J]. 钻井液与完井液,2020,37(3):377–383. LIU Jiankun, JIANG Tingxue, BIAN Xiaobing, et al. The countermeasure of low cost and high efficiency fracturing technology of normal pressure shale gas[J]. Drilling Fluid & Completion Fluid, 2020, 37(3): 377–383.
[19]	TADA H, PARIS P C, IRWIN G R. The stress analysis of cracks handbook[M]. 3rd ed. New York: ASME Press, 2000.
[20]	HSU Y C. The infinite sheet with two radial cracks from cylindrical hole under inclined tension or in-plane shear[J]. International Journal of Fracture, 1977, 13(6): 839–845. doi: 10.1007/BF00034326
[21]	唐世斌,刘向君,罗江,等. 水压诱发裂缝拉伸与剪切破裂的理论模型研究[J]. 岩石力学与工程学报,2017,36(9):2124–2135. TANG Shibin, LIU Xiangjun, LUO Jiang, et al. Theoretical model for tensile and shear crack initiation at the crack tip in rock subjected to hydraulic pressure[J]. Chinese Journal of Rock Mechanics and Engineering, 2017, 36(9): 2124–2135.
[22]	唐世斌,张恒. 基于最大周向拉应变断裂准则的岩石裂纹水力压裂研究[J]. 岩石力学与工程学报,2016,35(增刊1):2710–2719. TANG Shibin, ZHANG Heng. Hydraulic fracture prediction theory based on the maximum tangential strain criterion[J]. Chinese Journal of Rock Mechanics and Engineering, 2016, 35(supplement 1): 2710–2719.
[23]	刘明明,马收,刘立之,等. 页岩气水平井压裂施工中暂堵球封堵效果研究[J]. 钻采工艺,2020,43(6):44–48. LIU Mingming, MA Shou, LIU Lizhi, et al. Study on the effect of temporary plugging ball in fracturing of horizontal shale gas well[J]. Drilling & Production Technology, 2020, 43(6): 44–48.
[24]	郭建春,赵峰,詹立,等. 四川盆地页岩气储层暂堵转向压裂技术进展及发展建议[J]. 石油钻探技术,2023,51(4):170–183. doi: 10.11911/syztjs.2023039 GUO Jianchun, ZHAO Feng, ZHAN Li, et al. Recent advances and development suggestions of temporary plugging and diverting fracturing technology for shale gas reservoirs in the Sichuan Basin[J]. Petroleum Drilling Techniques, 2023, 51(4): 170–183. doi: 10.11911/syztjs.2023039
[25]	吕振虎,吕蓓,罗垚,等. 基于光纤监测的段内多簇暂堵方案优化[J]. 石油钻探技术,2024,52(1):114–121. LYU Zhenhu, LYU Bei, LUO Yao, et al. Optimization of in-stage multi-cluster temporary plugging scheme based on optical fiber monitoring[J]. Petroleum Drilling Techniques, 2024, 52(1): 114–121.
[26]	蒋廷学. 非常规油气藏新一代体积压裂技术的几个关键问题探讨[J]. 石油钻探技术,2023,51(4):184–191. doi: 10.11911/syztjs.2023023 JIANG Tingxue. Discussion on several key issues of the new-generation network fracturing technologies for unconventional reser-voirs[J]. Petroleum Drilling Techniques, 2023, 51(4): 184–191. doi: 10.11911/syztjs.2023023
[27]	吴峙颖,路保平,胡亚斐,等. 压裂多级裂缝内动态输砂物理模拟实验研究[J]. 石油钻探技术,2020,48(4):106–110. WU Shiying, LU Baoping, HU Yafei, et al. Experimental study on the physical simulation of dynamic sand transport in multi-stage fractures[J]. Petroleum Drilling Techniques, 2020, 48(4): 106–110.
[28]	任洪达,董景锋,高靓,等. 新疆油田玛湖砂岩储层自悬浮支撑剂现场试验[J]. 油气藏评价与开发,2023,13(4):513–518. REN Hongda, DONG Jingfeng, GAO Jing, et al. Field test of self-suspending proppant at Mahu sandstone reservoir in Xinjiang Oilfield[J]. Petroleum Reservoir Evaluation and Development, 2023, 13(4): 513–518.
[29]	郭建春,路千里,何佑伟. 页岩气压裂的几个关键问题与探索[J]. 天然气工业,2022,42(8):148–161. GUO Jianchun, LU Qianli, HE Youwei. Key issues and explorations in shale gas fracturing[J]. Natural Gas Industry, 2022, 42(8): 148–161.
[30]	曾波,冯宁鑫,姚志广,等. 深层页岩气储层水力压裂裂缝扩展影响机理[J]. 断块油气田,2024,31(2):246–256. ZENG Bo, FENG Ningxin, YAO Zhiguang, et al. Influence mechanism of hydraulic fracturing fracture propagation in deep shale gas reservoirs[J]. Fault-Block Oil & Gas Field, 2024, 31(2): 246–256.
[31]	杨兆中,袁健峰,张景强,等. 四川盆地海相页岩水平井压裂裂缝研究进展及认识[J]. 油气藏评价与开发,2024,14(4):600–609. YANG Zhaozhong, YUAN Jianfeng, ZHANG Jingqiang, et al. Research progress and understanding of fracturing fractures in horizontal wells of marine shale in Sichuan Basin[J]. Petroleum Reservoir Evaluation and Development, 2024, 14(4): 600–609.

施引文献(35)

期刊类型引用(25)

1.	杨斌，张浩，杨建，李越，曾港彬，刘国庆，杨珊. 基于裂缝亚临界扩展理论的页岩吸水起裂微观力学机制. 天然气工业. 2025(02): 105-113 . 百度学术
2.	杨坤. 塔西南山前破碎性地层安全高效钻井液关键技术. 化工管理. 2025(04): 165-168 . 百度学术
3.	智慧文，栗涵洁，权子涵. 井研地区筇竹寺组页岩储层水平井钻井井壁稳定性评价. 中外能源. 2024(03): 62-66 . 百度学术
4.	杨斌，许成元，张浩，郭予凡，杨建，李越，赵建国. 深部破碎地层井壁失稳机理研究进展与攻关对策. 石油学报. 2024(05): 875-888 . 百度学术
5.	衣方宇，王琦，杨杰，朱子阳，王昶皓，庞皓安. 苏里格气田多层理泥岩井壁坍塌机理分析. 能源与环保. 2024(05): 97-104 . 百度学术
6.	王帅，谢勰，刘厚彬，陶思才，杜爽，冷程锦，冯春宇. 川南龙马溪组页岩地层井壁崩塌破坏机制研究. 钻采工艺. 2024(05): 33-40 . 百度学术
7.	祁文莉，吴惠梅，谢贤东，楼一珊，刘宏. 苏北盆地地层岩石特性及井壁坍塌周期研究. 中国海上油气. 2024(06): 108-118 . 百度学术
8.	苏广，李刚权，赵志良，陈忠云，叶寒，邓富元. 考虑化学势作用下钻井液侵入泥页岩水层理论模型. 山东石油化工学院学报. 2024(04): 62-67 . 百度学术
9.	刘卫彬，徐兴友，陈珊，白静，李耀华. 松辽盆地陆相页岩油地质工程一体化高效勘查关键技术与工程示范. 地球科学. 2023(01): 173-190 . 百度学术
10.	施赵南，高斐. 力-化耦合作用下水化坍塌周期研究. 科技创新与应用. 2023(13): 71-74 . 百度学术
11.	张文，刘向君，梁利喜，熊健. 致密砂岩地层气体钻井井眼稳定性试验研究. 石油钻探技术. 2023(02): 37-45 . 本站查看
12.	黄亮，余意，任冠龙，孟文波，郑文培. 裸眼测试井壁失稳概率及参数敏感性分析. 石油钻采工艺. 2023(02): 143-150 . 百度学术
13.	喻贵民. 一种颗粒离散元的井壁稳定性分析模型. 石油钻采工艺. 2023(02): 129-135 . 百度学术
14.	王丽君. 苏里格硬脆性泥岩地层井壁失稳机制及钻井液技术对策. 精细石油化工进展. 2023(04): 21-25 . 百度学术
15.	丁乙，雷炜，刘向君，秦章晋，梁利喜，周吉羚，熊健. 页岩气储层自吸–水化损伤–离子扩散相关性试验研究. 石油钻探技术. 2023(05): 88-95 . 本站查看
16.	宋先知，郭勇，向冬梅，鞠鹏飞，谭强，刘伟. 呼图壁背斜水基钻井液井壁失稳机理多场耦合分析. 新疆石油天然气. 2023(04): 1-9 . 百度学术
17.	张震，万秀梅，吴鹏程，李郑涛，文莉. 川南龙马溪组深层页岩井壁失稳原因分析及对策. 特种油气藏. 2022(01): 160-168 . 百度学术
18.	王伟吉，李大奇，金军斌，徐江，张杜杰. 顺北油气田破碎性地层井壁稳定技术难题与对策. 科学技术与工程. 2022(13): 5205-5212 . 百度学术
19.	胡清富，刘春来，牟少敏，李增乐，司小东. 伊拉克东巴油田Tanuma组泥页岩高效防塌钻井液技术. 石油钻探技术. 2022(04): 76-82 . 本站查看
20.	聂岚，刘其明，李衡. 川西南资阳—东峰场区块须家河组地层井壁稳定性. 天然气技术与经济. 2022(04): 49-53+59 . 百度学术
21.	刘卫彬，徐兴友，张君峰，陈珊，白静，刘畅，李耀华. 陆相页岩地层地质-工程一体化水平井精确钻探技术——以松辽盆地吉页油1HF井为例. 中国地质. 2022(06): 1808-1822 . 百度学术
22.	薛少飞，李伟，张文哲，王波，殷嘉伟. 延长区块炭质泥岩高性能水基钻井液研究. 辽宁化工. 2021(05): 720-722 . 百度学术
23.	程万，孙家应，尹德战，蒋国盛. 深层泥页岩井壁失稳机理与预测模型研究进展. 钻探工程. 2021(10): 21-28 . 百度学术
24.	刘浩童，刘玉栋，段晓苗，王楠，李玲，潘晨. 页岩稳定剂氧化石墨烯的研制与评价. 断块油气田. 2021(06): 765-768+791 . 百度学术
25.	金军斌，欧彪，张杜杰，王希勇，李大奇，王逸. 深部裂缝性碳酸盐岩储层井壁稳定技术研究现状及展望. 长江大学学报(自然科学版). 2021(06): 47-54 . 百度学术

其他类型引用(10)

资源附件(0)

图(14) / 表(3)

计量

文章访问数: 42
HTML全文浏览量: 4
PDF下载量: 7
被引次数: 35

1. 层理页岩水平井坍塌压力预测模型
1.1 考虑水化应变作用的井周应力
1.2 原地应力产生的井周应力
1.3 井眼总有效应力
1.4 坍塌压力的确定
2. 坍塌压力模型求解
3. 力化耦合下层理性页岩井壁失稳影响因素分析
3.1 地应力机制对坍塌压力的影响
3.2 层理面产状对坍塌压力的影响
3.3 含水量对坍塌压力的影响
3.4 水化时间对坍塌压力的影响
4. 结论及建议

1. 层理页岩水平井坍塌压力预测模型
1.1 考虑水化应变作用的井周应力
1.2 原地应力产生的井周应力
1.3 井眼总有效应力
1.4 坍塌压力的确定
2. 坍塌压力模型求解
3. 力化耦合下层理性页岩井壁失稳影响因素分析
3.1 地应力机制对坍塌压力的影响
3.2 层理面产状对坍塌压力的影响
3.3 含水量对坍塌压力的影响
3.4 水化时间对坍塌压力的影响
4. 结论及建议

参考文献(31)

施引文献(35)

资源附件(0)

南川页岩气田压裂工艺改进及应用

作者简介: 周成香（1985—），湖北老河口人，2009年毕业于长江大学石油工程专业，2016年获中国石油大学（北京）石油与天然气工程专业硕士学位，高级工程师，主要从事页岩气勘探开发方面的研究工作。E-mail：278738690@qq.com

计量

出版历程

The Improvement and Application of Fracturing Technology in the Nanchuan Shale Gas Field

1. 层理页岩水平井坍塌压力预测模型

1.1 考虑水化应变作用的井周应力

1.2 原地应力产生的井周应力

1.3 井眼总有效应力

1.4 坍塌压力的确定

2. 坍塌压力模型求解

3. 力化耦合下层理性页岩井壁失稳影响因素分析

3.1 地应力机制对坍塌压力的影响

3.2 层理面产状对坍塌压力的影响

3.3 含水量对坍塌压力的影响

3.4 水化时间对坍塌压力的影响

4. 结论及建议

期刊类型引用(25)

其他类型引用(10)

计量

出版历程

目录

1. 层理页岩水平井坍塌压力预测模型

1.1 考虑水化应变作用的井周应力

1.2 原地应力产生的井周应力

1.3 井眼总有效应力

1.4 坍塌压力的确定

2. 坍塌压力模型求解

3. 力化耦合下层理性页岩井壁失稳影响因素分析

3.1 地应力机制对坍塌压力的影响

3.2 层理面产状对坍塌压力的影响

3.3 含水量对坍塌压力的影响

3.4 水化时间对坍塌压力的影响

4. 结论及建议

作者简介:
周成香（1985—），湖北老河口人，2009年毕业于长江大学石油工程专业，2016年获中国石油大学（北京）石油与天然气工程专业硕士学位，高级工程师，主要从事页岩气勘探开发方面的研究工作。E-mail：278738690@qq.com