Well Depth Measured with MWD Error Correction and Calculation of Borehole Position Uncertainty
-
摘要:
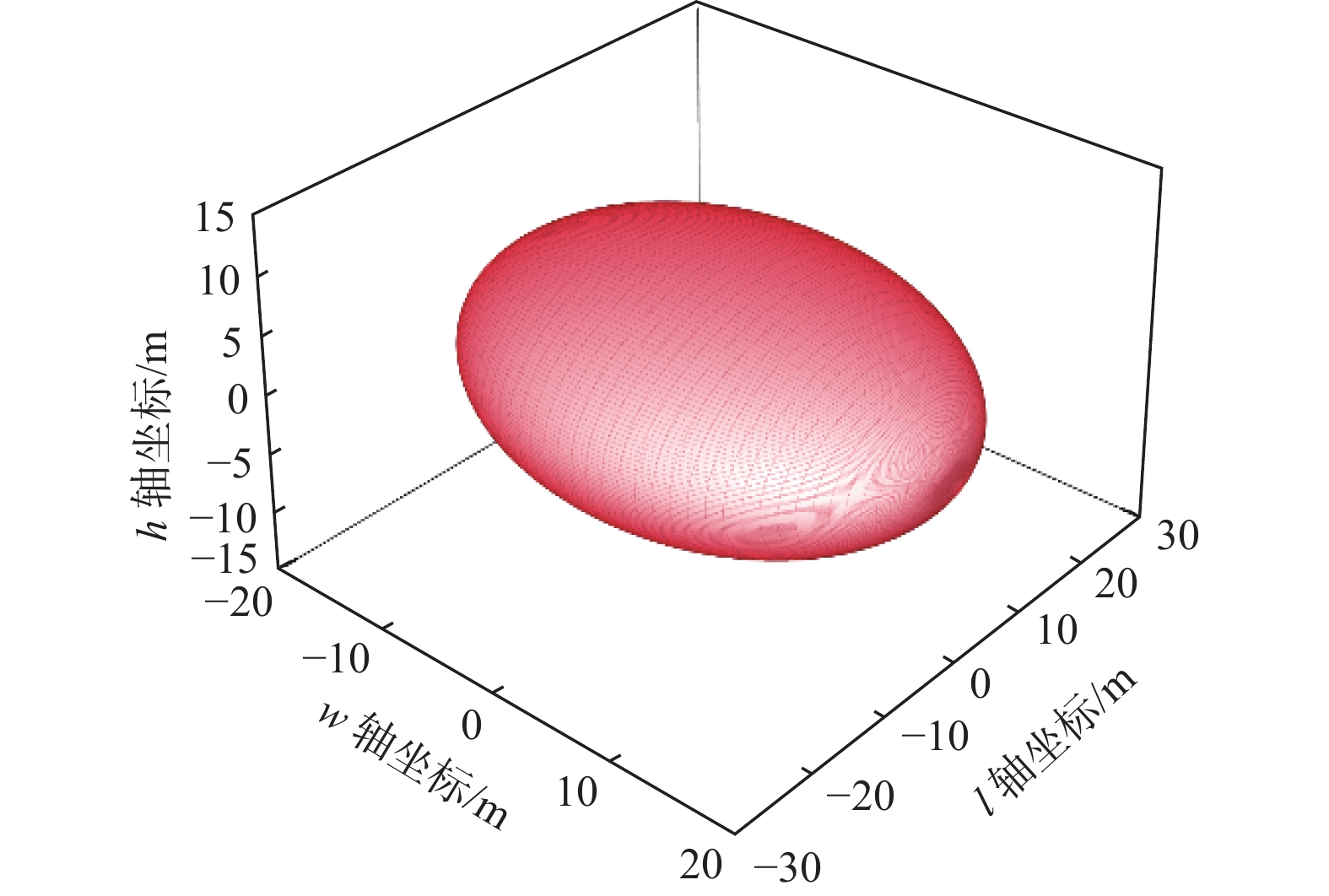
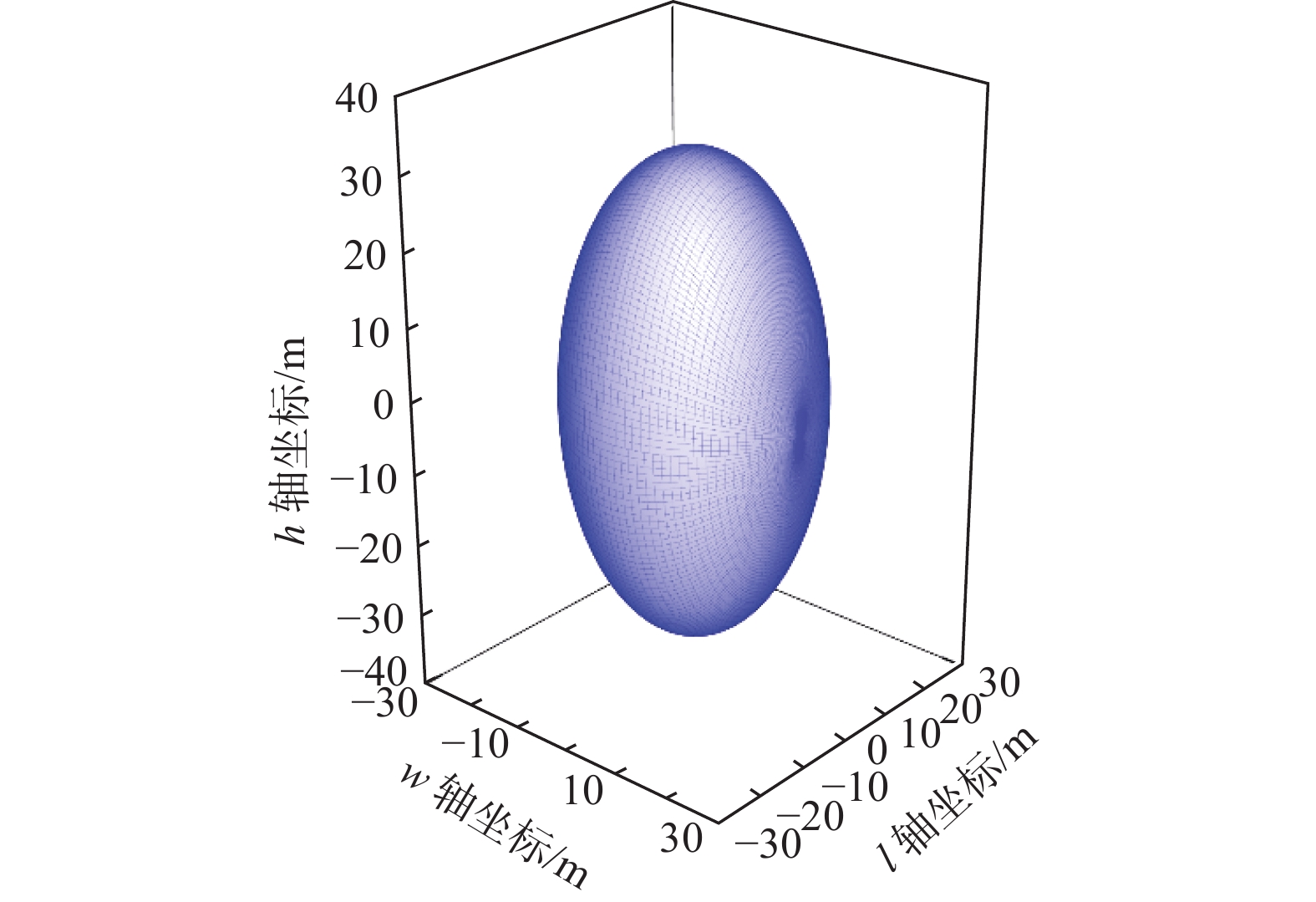
深井和超深井钻井过程中井下温度高、井内钻具承受的拉力大，导致随钻测量的井深误差较大。为此，考虑不同井深处井内温度、热膨胀系数、钻具轴向力和钻具规格等因素的影响，在测点处对井内钻具分段，结合井下温度随钻测量结果和井内钻具受力分析结果，建立了随钻测量井深的热膨胀校正模型和弹性拉伸校正模型，以及计算热膨胀校正误差限和弹性拉伸校正误差限的模型，并给出了随钻测量井深热膨胀和弹性拉伸校正后的井眼位置不确定性计算方法。实例计算表明，超深井钻井过程中由热膨胀和弹性拉伸导致的井内钻具伸长量可达10 m以上；随钻测量井深进行热膨胀和弹性拉伸校正后，可以显著减小测点垂深误差和误差椭球的大小。研究结果为提高井深随钻测量精度与科学计算井眼位置不确定性提供了理论依据。
Abstract:When drilling deep and ultra-deep wells, the high downhole temperature and the large tensile force on the drilling tools in the well result in a large well depth error measured with measurement with drilling (MWD). Therefore, the influence of downhole temperature, thermal expansion coefficient, axial force of drilling tools, and specifications of drilling tools in different well depths was considered, and the drilling tools in the well were segmented at the measurement point. According to the results of the downhole temperature measured with MWD and the force analysis of drilling tools in the well, models of thermal expansion and elastic tension correction for the well depth measured with MWD were established. In addition, the models for the calculation of the error limits of the thermal expansion correction and the elastic tension correction, as well as the calculation method of wellbore position uncertainty after correcting thermal expansion and elastic tension errors of measured well depth with MWD were given. The example calculations demonstrate that during the drilling of ultra-deep wells, the elongation of drilling tools in the well caused by thermal expansion and elastic tension can reach more than 10 m. By correcting the thermal expansion and elastic tension of the measured depth with MWD, the vertical depth errors and the size of the error ellipsoid of the measurement points can be significantly reduced. The results provide a theoretical basis for improving the accuracy of well depth measured with MWD and scientific calculation of wellbore position uncertainty.
-
-
![]()
图 1 测点编号与井内钻具分段编号
Figure 1. Numbers of survey points and drilling tool sections in a well
![]()
图 3 井内钻具承受的轴向力随井深的变化曲线
Figure 3. Variation of axial force on the drilling tool in a well with well depth
表 1 井眼轨迹参数的部分数据
Table 1 Partial data of wellbore trajectory parameters
井深/m 井斜角/(°) 方位角/(°) 垂深/m 北坐标/m 东坐标/m 0 0 0 0 0 0 7 900.00 0 0 7 900.00 0 0 7 905.00 1.33 110.92 7 905.00 −0.02 0.05 7 920.00 5.33 110.92 7 919.97 −0.33 0.87 7 950.00 13.33 110.92 7 949.55 −2.07 5.41 7 980.00 21.33 110.92 7 978.16 −5.26 13.75 8 010.00 29.33 110.92 8 005.26 −9.84 25.73 8 040.00 37.33 110.92 8 030.30 −15.72 41.12 8 070.00 45.33 110.92 8 052.81 −22.79 59.61 8 100.00 53.33 110.92 8 072.34 −30.90 80.85 8 130.00 61.33 110.92 8 088.52 −39.91 104.42 8 160.00 69.33 110.92 8 101.04 −49.64 129.86 8 179.12 74.43 110.92 8 106.98 −56.13 146.83 ⋮ ⋮ ⋮ ⋮ ⋮ ⋮ 8 454.91 74.43 110.92 8 181.01 −150.99 394.99 
下载: 导出CSV
表 2 钻具参数
Table 2 Parameters of drilling tools
钻具 长度/m 外径/mm 内径/mm PDC钻头 0.30 149.3 螺杆 9.20 120.0 44.50 无磁钻铤 9.00 120.0 63.50 无磁短节 1.00 120.0 63.50 浮阀 0.40 127.0 50.80 加重钻杆 10.00 88.9 52.40 ϕ88.9 mm钻杆 10.00 88.9 70.21 ϕ114.3 mm钻杆 10.00 114.3 97.18
下载: 导出CSV
-
[1] 于瑞丰,刁斌斌,高德利. 考虑磁方位校正的井眼轨迹测量误差计算[J]. 石油钻探技术,2023,51(6):25–31. YU Ruifeng, DIAO Binbin, GAO Deli. Calculation for wellbore trajectory measurement error incorporating magnetic azimuth correction[J]. Petroleum Drilling Techniques, 2023, 51(6): 25–31.
[2] 孟庆威,姜天杰,刘泳敬,等. 基于有限元分析的方位角误差计算和修正[J]. 石油钻探技术,2022,50(3):66–73. MENG Qingwei, JIANG Tianjie, LIU Yongjing, et al. Calculation and correction of azimuth errors based on finite element analysis[J]. Petroleum Drilling Techniques, 2022, 50(3): 66–73.
[3] 孟卓然. BHA轴向磁干扰对方位测量误差的影响:基于人工磁场模拟方法[J]. 石油学报,2020,41(8):1011–1018. MENG Zhuoran. Effect of BHA axial magnetic interference on the azimuth measurement error: a simulation method based on artificial magnetic field[J]. Acta Petrolei Sinica, 2020, 41(8): 1011–1018.
[4] 刘建光,底青云,张文秀. 基于多测点分析法的水平井高精度磁方位校正方法[J]. 地球物理学报,2019,62(7):2759–2766. LIU Jianguang, DI Qingyun, ZHANG Wenxiu. High precision magnetic azimuth correction of horizontal well by multi-station analysis[J]. Chinese Journal of Geophysics, 2019, 62(7): 2759–2766.
[5] 范光第,蒲文学,赵国山,等. 磁力随钻测斜仪轴向磁干扰校正方法[J]. 石油钻探技术,2017,45(4):121–126. FAN Guangdi, PU Wenxue, ZHAO Guoshan, et al. Correction methods for axial magnetic interference of the magnetic inclinometer while drilling[J]. Petroleum Drilling Techniques, 2017, 45(4): 121–126.
[6] 许昊东,黄根炉,张然,等. 磁力随钻测量磁干扰校正方法研究[J]. 石油钻探技术,2014,42(2):102–106. XU Haodong, HUANG Genlu, ZHANG Ran, et al. Method of magnetic interference correction in survey with magnetic MWD[J]. Petroleum Drilling Techniques, 2014, 42(2): 102–106.
[7] WILSON H, LOFTS J C, PAGE G C, et al. Depth control: reconciliation of LWD and wireline depths, standard practice and an alternative simple but effective method[R]. SPE 89899, 2004.
[8] CHIA C R, LAASTAD H, KOSTIN A, et al. A new method for improving LWD logging depth[R]. SPE 102175, 2006.
[9] PEDERSEN B K, CONSTABLE M V. Operational procedures and methodology for improving LWD and wireline depth control, Kristin Field, Norwegian Sea[J]. Petrophysics, 2007, 48(2): 90–103.
[10] BOLT H. A method for determining well depth: PCT/GB2018/000030[P]. 2018-08-30.
[11] 卿元华,李秀彬. 钻具深度与测井深度误差的成因探讨[J]. 西部探矿工程,2016,28(6):25–28. QING Yuanhua, LI Xiubin. Discussion on the cause of the error of drilling depth and logging depth[J]. West-China Exploration Engineering, 2016, 28(6): 25–28.
[12] 郭骁,李思洋,李红星,等. 井深误差对井眼轨迹不确定性影响及校正[J]. 天然气与石油,2020,38(3):79–84. GUO Xiao, LI Siyang, LI Hongxing, et al. The impact of well depth error on wellbore trajectory uncertainties and error correction[J]. Natural Gas and Oil, 2020, 38(3): 79–84.
[13] ISCWSA. Introduction to wellbore positioning[M/OL]. Inverness: University of the Highlands and Islands, 2012[2024-02-04]. https://www.iscwsa.net/media/files/page/f1c1e97e/introduction-to-wellbore-positioning-ebook-v9-10-2017.pdf.
[14] 刘修善. 井眼轨迹不确定性表征方法[J]. 石油勘探与开发,2019,46(2):391–396. LIU Xiushan. Borehole trajectory uncertainty and its characterization[J]. Petroleum Exploration and Development, 2019, 46(2): 391–396.
[15] WILLIAMSON H S. Accuracy prediction for directional MWD[R]. SPE 56702, 1999.
[16] EKSETH R. Uncertainties in connection with the determination of wellbore positions[D]. Trondheim: Norwegian University of Science and Technology, 1998.
[17] 唐海雄,张俊斌,汪顺文,等. 高温致测试管柱伸长和受力计算分析[J]. 石油机械,2010,38(5):84–86. TANG Haixiong, ZHANG Junbin, WANG Shunwen, et al. Calculation and analysis of elongation and force of pipe string induced by high temperature[J]. China Petroleum Machinery, 2010, 38(5): 84–86.
[18] SY/T 5435—2012 定向井轨道设计与轨迹计算[S]. SY/T 5435—2012 Well path planning and survey calculation for directional drilling[S].
[19] 韩志勇. 定向钻井设计与计算[M]. 2版. 东营:中国石油大学出版社,2007:48-49. HAN Zhiyong. Directional drilling design and calculation[M]. 2nd ed. Dongying: China University of Petroleum Press, 2007: 48-49.
[20] FULLER H Q. Physics, including human applications[M]. New York: Harper & Row, 1978: 281-284.
[21] LUBINSKI A. A study of the buckling of rotary drilling strings[J]. API Drilling and Production Practice, 1950, 11(2): 178–214.
[22] 高德利. 油气井管柱力学与工程[M]. 东营:中国石油大学出版社,2006:80-88. GAO Deli. Down-hole tubular mechanics and its applications[M]. Dongying: China University of Petroleum Press, 2006: 80-88.
[23] WILLIAMSON H S. Accuracy prediction for directional measurement while drilling[J]. SPE Drilling & Completion, 2000, 15(4): 221–233.
-
期刊类型引用(11)
1. 赖书斌，王宏，王军. CMT增材再制造含预制槽30CrMo板组织性能及热处理研究. 机电工程技术. 2025(01): 118-122 . 
百度学术
2. 徐斌荣，王军，罗震，张小龙，刘宏亮，郭龙龙. 预制槽尺寸对30CrMo板再制造残余应力的影响. 焊接. 2024(08): 22-30 . 百度学术
3. 郭龙龙，王军，徐斌荣，刘春伟，付堃，隽鸿科，耿东恒，王宏. 30CrMo板CMT再制造熔覆层成形及组织性能. 精密成形工程. 2023(08): 99-105 . 百度学术
4. 郭龙龙，王军，刘宏亮，刘广阔，徐林，曹嘉晨. 30CrMo板增材再制造的温度场及残余应力. 船舶工程. 2023(06): 140-148 . 百度学术
5. 邹山梅，赵丽丽. 金属加工设备防腐防护外涂层的应用现状研究. 世界有色金属. 2022(02): 49-51 . 百度学术
6. 赵传伟，刘晗，吴仲华，霍卫东，王可可，陈锐，王卫. 双层套管段铣工具研制. 石油机械. 2022(11): 43-49 . 百度学术
7. 苗娟，黄兵，谢力，汤明. 高钢级套管段铣工具优化及性能评价. 特种油气藏. 2021(02): 163-170 . 百度学术
8. 万春燕，樊春明，贾向锋，刘文霄，孙传轩，刘兴铎. 水下采油树用闸阀和驱动器试验技术研究. 石油矿场机械. 2021(04): 25-30 . 百度学术
9. 李莹莹，任美鹏，王名春，南有博，吴沿，王秀通. 海洋环境水下采油树腐蚀与防护研究进展. 装备环境工程. 2021(07): 130-139 . 百度学术
10. 颜帮川，董长银，黄亮，魏安超，闫切海，方达科，钟奕昕，王力智. 南海高温高压气藏机械防砂筛管动态腐蚀评价实验研究. 特种油气藏. 2020(03): 148-156 . 百度学术
11. 魏军会，杜文波，张宝，崔佳凯，易俊，康诗琪，李建明，冯春宇. 焊道间隔冷却时间对Inconel 625温度场的影响. 兵器材料科学与工程. 2020(06): 90-94 . 百度学术
其他类型引用(5)
计量
- 文章访问数: 168
- HTML全文浏览量: 27
- PDF下载量: 100
- 被引次数: 16
