Numerical Simulation of Shale Oil Well Productivity Based on Shale Oil-Water Two-Phase Flow Characteristics
-
摘要:
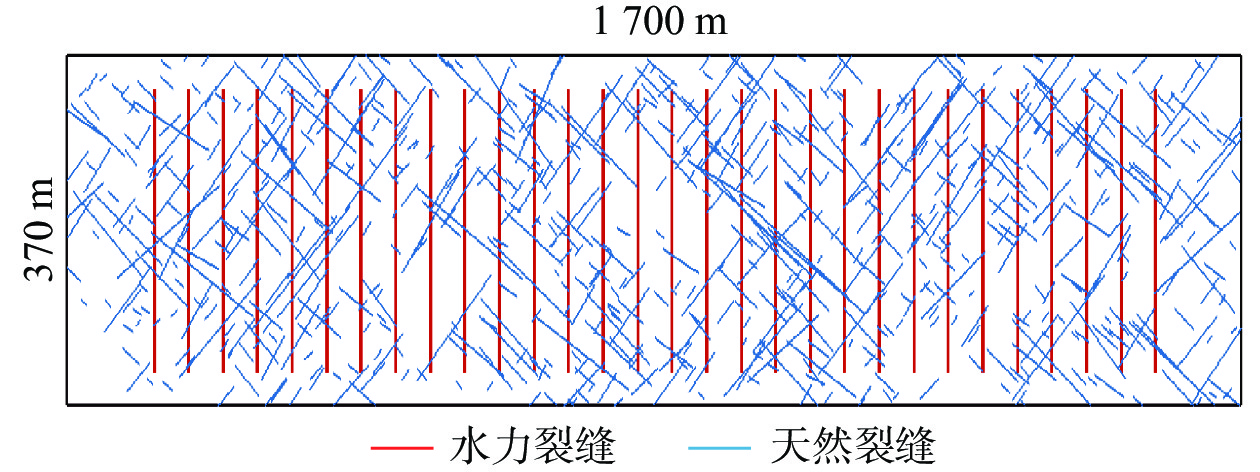
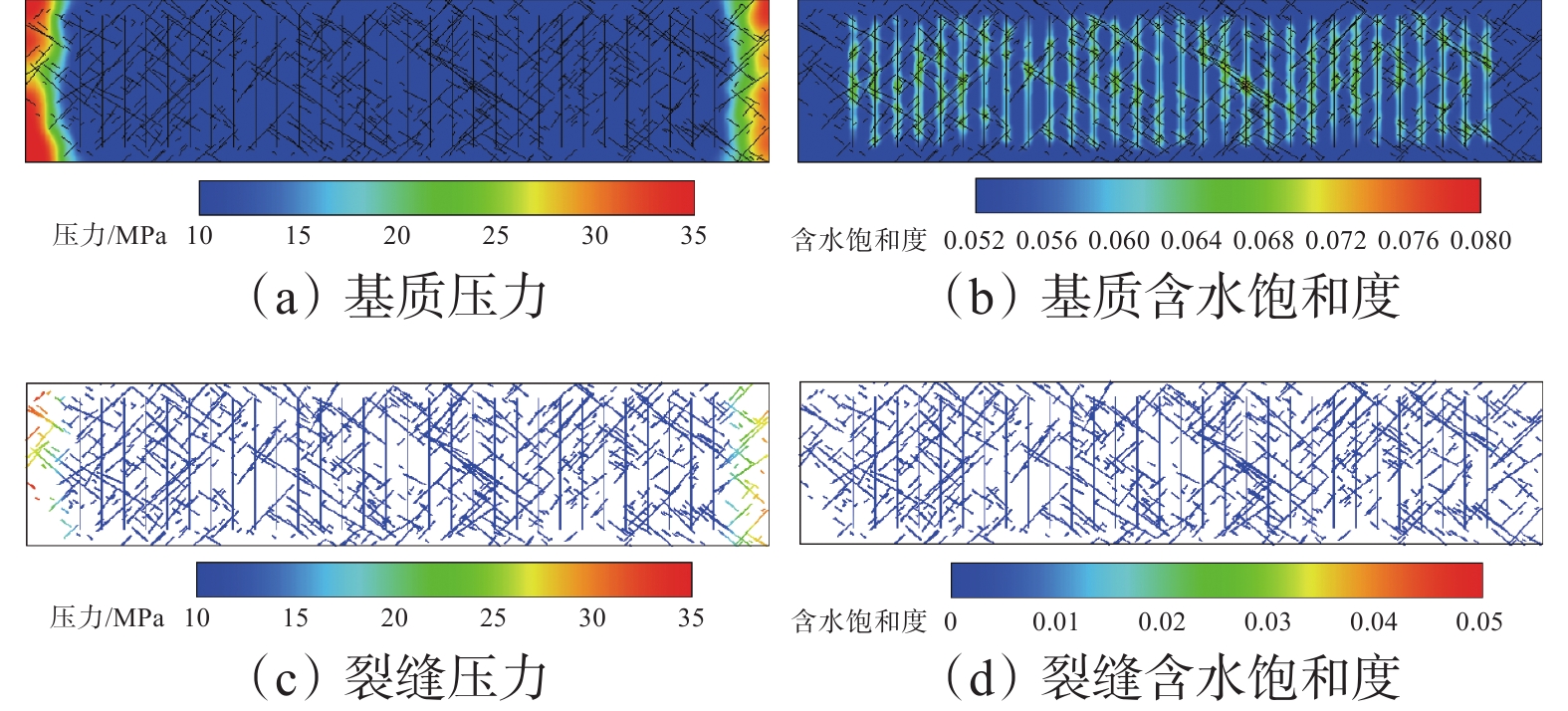
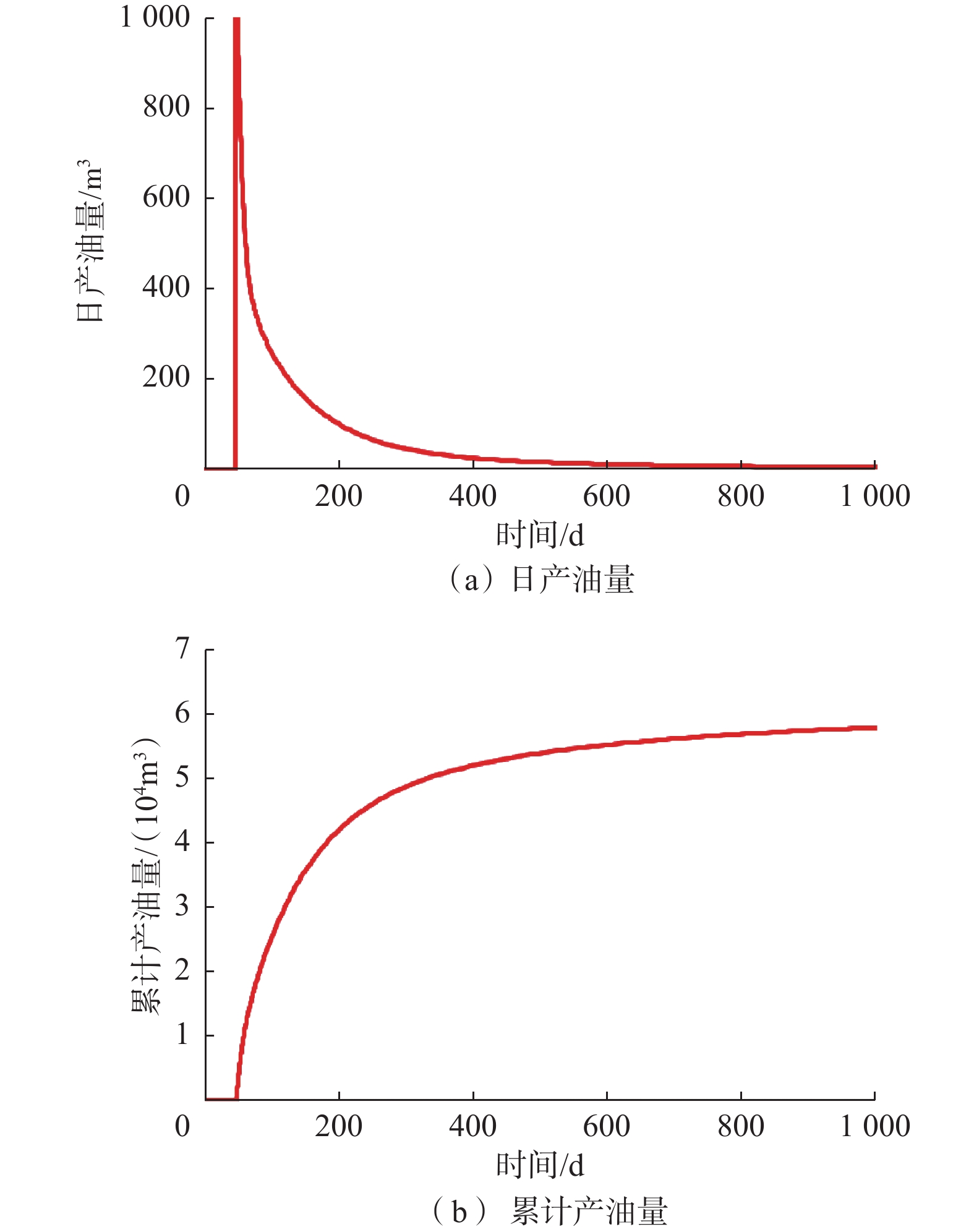
页岩孔隙结构及固液相互作用复杂，其微观渗流特性加大了页岩油产能预测的难度。为准确评价体积压裂后多尺度孔隙结构发育的页岩油藏产能，基于页岩储层油水两相相渗计算方法和嵌入式离散裂缝模型，考虑页岩真实孔隙结构作用下的微观油水两相渗流特性，形成了考虑页岩体积压裂页岩油藏产能的数值模拟方法。基于页岩储层孔径分布计算油水相渗曲线，结合页岩油藏压裂/生产流程，开展了页岩油藏压裂液空间分布以及油井产能评价模拟分析。结果表明，不同孔径分布下的页岩油水两相相渗曲线存在差异，压裂液主要分布在压裂裂缝、与其相连的天然裂缝以及其周边基质中，在闷井过程中裂缝内压裂液逐渐渗吸进入基质并置换基质中原油，经体积压裂可实现改造区域的整体动用。研究结果可以从微观油水两相渗流特性与宏观产能评价角度为页岩油藏高效开发提供技术支撑。
Abstract:The pore structure of shale is complex, and solid-liquid interaction occurs. In addition, its microscopic flow characteristics increase the difficulty of shale oil productivity prediction. In order to accurately evaluate the productivity of shale oil reservoirs with multi-scale pore structures after volume fracturing, the microscopic multi-phase flow characteristics under the action of real pore structure of shale were considered based on the oil-water two-phase relative permeability calculation method and embedded discrete fracture model (EDFM) of shale reservoirs. As a result, a numerical simulation method for shale oil reservoir productivity considering shale volume fracturing was developed. The oil-water two-phase relative permeability curve was calculated based on the pore size distribution of shale reservoirs, and combined with the fracturing/production process of shale reservoirs, the spatial distribution of fracturing fluid in shale oil reservoirs and the productivity evaluation of oil well were simulated and analyzed. The results show that there are differences in the oil-water two-phase relative permeability curves of shale under different pore size distributions. Fracturing fluids are mainly distributed in fracturing fractures, natural fractures connected with them, and the surrounding matrix. During the process of shut-in, the fracturing fluid in the fracture is gradually imbibed into the matrix, displacing the crude oil in the matrix and realizing the whole utilization of the stimulated area by volume fracturing. The research results can provide technical support for the efficient development of shale oil reservoirs from the perspective of microscopic oil-water two-phase flow characteristics and macroscopic productivity evaluation.
-
塔河油田碳酸盐岩缝洞型油藏与普通砂岩油藏不同，储集空间类型多样、形态差异较大，非均质性极强[1]。该油田开发初期主要依靠天然能量开采，随着开发不断进行，天然能量出现不足，采出能力开始下降，注水开发是初期解决该问题的最有效方法，但进入注水开发后期，储层经过长时间注水后，油水界面升高，驱油效果逐渐变差[2-6]。为此，进行了碳酸盐岩缝洞型油藏气水复合驱技术研究。该技术是在长时间注水后，改为注入氮气，注入的氮气会聚集在储集体高部位的阁楼体内[5-8]，将阁楼体内的剩余油油置换出来，但是由于缺乏横向驱动力，剩余油可能会大量富集在注采井网的井间；于是，在当前注气井网条件下，需再次注水增加横向水驱动力，提高井间剩余油的动用程度，从而改善碳酸盐岩缝洞型油藏的开发效果。该技术在塔河油田 4 区 7 个注采井组进行了现场应用，并获得良好的增产效果。
1. 碳酸盐岩缝洞型油藏特征
塔河油田奥陶系碳酸盐岩缝洞型油藏发育于新疆塔里木盆地沙雅隆起阿克库勒凸起的西南部，油藏埋深5 400.00～7 600.00 m，储集空间主要为溶蚀孔洞、大型洞穴和溶蚀裂缝，储集体主要为裂缝–溶洞型和裂缝–孔洞型，部分区域奥陶系一间房组地层发育微裂缝[9]。其中洞穴和孔洞的储集性能最好，裂缝既是储集空间，又是流体流动的主要通道，流体流动以管流为主[10-11]。各类岩溶体储层空间展布具有极强的非均质性，油气水运移规律复杂。
2. 气水复合驱油开发模式研究
2.1 气水复合驱油机理
在利用多属性地震资料描述储集体形态特征的基础上，通过刻蚀玻璃的方法建立了一套20 mm×30 mm的缝洞储集体物理模拟模型，该模型设计为裂缝–溶洞型储集体，孔隙度为18%。利用该模型进行气水复合驱油物理模拟试验，先注入试验用油，待模型空腔充满试验用油，再注水进行水驱，待出口已经完全出水后再注入氮气，注入一定量氮气后再次注水。试验过程中观察不同阶段模型内流体的运移情况，结果见图1。从图1可以看出，该模型在充满试验用油经水驱后，顶部的7、8号储集体内仍存在大量剩余油（见图1（a））；对其进行气驱，7、8号储集体内的剩余油被驱替到水驱通道上（见图1（b）），再次进行水驱，注入水将水驱通道上的剩余油从出口端驱替出（见图1（c））。由此可知缝洞型油藏气水复合驱油机理：氮气作为纵向驱动力，向下驱替缝洞体顶部剩余油，将剩余油驱替至水驱通道上，注入水作为横向驱动力，形成二次水驱。
![]()
图 1 复杂缝洞模型气水复合驱油流体运移情况Figure 1. Fluids migration of gas-water composite flooding in a complex fracture-cave model2.2 气水复合驱开发方式
在认识气水复合驱油机理的基础上，利用地震资料刻画井洞关系，根据生产动态识别注采井之间的连通通道，明确剩余油分布，针对不同剩余油分布特征构建了4种井组模式（见图2）：对于水驱通道在含油高度内的“阁楼油”，构建了注入井先注气、后注水的常规协同模式，为单方向一注一采的模式；对于水驱失效的多井区域的“阁楼油”，构建了注入井注气、周边邻井注水的栅状协同模式；对于出现气窜的井组，构建了换向协同模式；对于失效或未见效并且水驱通道在含油高度外的“阁楼油”，构建了注入井注气后先调流封堵水通道、再注水的调剖协同模式。
2.3 气水复合驱井网设计
主要依托岩溶背景及储层展布特征，根据基础井组模式有针对性地构建气水复合立体井网，如图3所示。对于风化壳岩溶，其展布面积广，多向连通条件好，构建面状注采井网；对于断溶体、古河道储层展布方向性强，连通特征表现为带状连通或线性连通，分别建立带状井网和线状井网[5]。
![]()
图 3 气水复合平面井网构建示意Figure 3. Schematic on the construction of the gas-water composite planar well pattern纵向上，根据井间通道路径长短、构造高低、规模大小等因素配置井网。对于有利驱替路径为陡构造、短路径、规模较小的山梁、断溶体或暗河等，如果采用低注高采井网很容易发生气窜，而采用高注低采井网则可以发挥作用集中、见效快和控制气窜的优势；有利驱替路径为缓构造、长路径，阁楼储集体靠近注入井，可以采用低注高采井网以提高驱替效率。
总体而言，需要根据通道的规模确定采用高注低采井网还是低注高采井网：短路径、小通道采用高注低采井网，以气驱为主，水驱为辅，以预防水窜；长路径、大通道采用低注高采井网，以水驱为主，气驱为辅，以提高气驱效率。实践中，2种纵向井网模式对不同规模的通道均有其优势。
2.4 气水复合驱参数设计
根据历史注水水驱效果确定水驱可动用空间，对比累计注气体积与水驱可动用空间判断通道内剩余油的再次充满程度，根据充满程度确定水驱历史等效阶段，用等效阶段的历史注水强度指导气水复合驱参数设计。
气水复合驱的作用过程分为2部分：1）垂向上，注入气将“阁楼油”驱至水驱可动用空间；2）横向上，注入水进入水驱可动用空间将油驱至受效井。注入气不断垂向驱油，关键是如何形成有效的横向水驱。根据历史注水水驱效果确定水驱可动用空间，通过对比累计注气体积与水驱可动用空间判断通道内剩余油的再次充满程度，根据剩余油充满程度确定水驱历史等效阶段，再根据等效阶段历史注水强度设计气水复合驱参数。理想驱替模型中，注采比应为1∶1，注入水前缘突破前的注水量等于增油量，注水过程中纵向上大量分水，少部分水形成了有效横向驱替。水驱结束时生产井总增油量即为有效横向水量，即水驱可驱扫空间总量。具体计算步骤（见图4）如下：
![]()
图 4 气水复合驱参数设计流程Figure 4. Flow chart of gas-water composite flooding parameters design1）确定水驱可动用空间体积。对于具有完整的水驱见效至失效阶段的注采井组，认为井间水驱可动用空间体积即水驱采油量的地下体积。
2）确定水驱可动用空间的充满程度。首先根据累计注入气量的地下体积与气驱采油量的地下体积的差，求出水驱通道中剩余油的体积；然后计算水驱可动空间的充满程度，即水驱通道剩余油体积与水驱可动用空间体积之比。
3）对应注水水驱等效阶段。利用等效原理，把任意气驱阶段对应的充满程度在水驱阶段找到对应相等充满程度的时间节点。
4）类比当时注水强度。通道充满程度相同时，注水受效日注水量为Qt。
5）确定目前的注水强度。设计目前的注水量QM≥Qt，即气水复合阶段要提供足够的横向驱动力驱动注入气顶替至水驱可动用空间内的剩余油，此时不需要考虑注水强度过大再次发生水窜的风险，因为单元注气阶段不同于注水水驱阶段，“阁楼油”可反复进入水驱通道。
3. 现场应用
气水复合驱技术在塔河油田4区7个注采井组进行了现场应用，均获得良好的增产效果，井组产油量平均提高86.0 t，累计增产油量1.3×104 t，且增油效果不断改善。下面以TK428CH–TK408井组为例，介绍气水复合驱技术的应用情况。
3.1 TK428CH–TK408井组概况
TK428CH井是注水兼注气井，TK408井是采油井，井组平面特征是沿山梁发育的风化壳岩溶，纵向特征为平缓山梁，注气路径长，“阁楼油”靠近注入井，采用低注高采井网（如图5所示）。
![]()
图 5 TK428CH–TK408井组地震属性资料与储集体刻画Figure 5. Seismic attribute data and reservoir bodies characterization of the TK428CH–TK408 well group3.2 注入参数设计
1）确定水驱可动用空间。水驱可动用空间等于前期受效增油量，该井组经历了完整的水驱阶段，水驱通道内的原油被驱替得较为彻底，因此该井组水驱增油量的地下体积等于水驱可动用空间的体积，通过计算该井组水驱可动用空间体积为5.47×104 m3。
2）判断水驱通道剩余油富集程度。该井累计注气5.70×104 m3，累计增油3.81×104 m3，通道内剩余油1.89×104 m3，水驱可动用空间充满程度为34.0%。
3）类比相同充满程度的水驱强度。当水驱阶段通道内剩余油充满程度为34.0%时，水驱处于效果变差阶段，此阶段注水量为300 m3/d，因此目前该井组合理注水量至少需要达到300 m3/d，连续注水。
4）现场注采调整及效果。调整前TK428CH井累计注气2.8×104 m3见效，后期效果出现变差趋势，计算水驱通道剩余油充满程度34.0%，水驱通道内仍富集大量剩余油，需要加强水驱动用水驱通道内的剩余油，于是TK428CH井恢复注水，并且将注水量提高至300 m3/d，TK408井生产效果改善，日增油量稳定在30 t。
4. 结论与建议
1）针对水驱和气驱无法有效动用塔河油田缝洞型碳酸盐岩油藏高部位剩余油的问题，根据其储层特征及剩余油分布特征，研究形成了气驱替油、水驱提供横向驱动力的气水复合驱技术。
2）现场应用表明，气水复合驱技术可以实现塔河油田缝洞型碳酸盐岩油藏高部位剩余油的有效动用，改善开发效果。
3）目前气水复合驱参数的设计是基于历史水驱效果进行的，还处于半定量阶段，建议进一步研究，通过地质建模和数值模拟实现定量计算。
-
![]()
图 2 两种典型页岩孔径分布概率曲线
Figure 2. Two typical probability curves for shale pore size distribution
![]()
图 3 两种孔径分布计算的油水两相相渗曲线
Figure 3. Oil-water two-phase relative permeability curves calculated with two pore size distributions
![]()
图 5 压裂结束时页岩油藏压力和含水饱和度分布模拟结果
Figure 5. Simulation results of pressure and water saturation distribution in shale oil reservoir after fracturing
![]()
图 6 闷井30 d后页岩油藏压力和含水饱和度分布模拟结果
Figure 6. Simulation results of pressure and water saturation distribution in shale oil reservoir after 30 days of shut-in
![]()
图 7 生产1 000 d后页岩油藏压力和含水饱和度分布模拟结果
Figure 7. Simulation results of pressure and water saturation distribution in shale oil reservoir after 1000 days of production
-
[1] 邹才能,马锋,潘松圻,等. 全球页岩油形成分布潜力及中国陆相页岩油理论技术进展[J]. 地学前缘,2023,30(1):128–142. ZOU Caineng, MA Feng, PAN Songqi, et al. Formation and distribution potential of global shale oil and the developments of continental shale oil theory and technology in China[J]. Earth Science Frontiers, 2023, 30(1): 128–142.
[2] 杨雷,金之钧. 全球页岩油发展及展望[J]. 中国石油勘探,2019,24(5):553–559. YANG Lei, JIN Zhijun. Global shale oil development and prospects[J]. China Petroleum Exploration, 2019, 24(5): 553–559.
[3] 佚名. 我国页岩油勘探开发在多领域获重要进展[J]. 天然气勘探与开发,2023,46(1):118. Anon. Shale oil exploration and development in China has made important progress in many fields[J]. Natural Gas Exploration and Development, 2023, 46(1): 118.
[4] 于学亮,胥云,翁定为,等. 页岩油藏 “密切割” 体积改造产能影响因素分析[J]. 西南石油大学学报(自然科学版),2020,42(3):132–143. YU Xueliang, XU Yun, WENG Dingwei, et al. Factors influencing the productivity of the multi-fractured shale oil reservoir with tighter clusters[J]. Journal of Southwest Petroleum University(Science & Technology Edition), 2020, 42(3): 132–143.
[5] 倪华峰,杨光,张延兵. 长庆油田页岩油大井丛水平井钻井提速技术[J]. 石油钻探技术,2021,49(4):29–33. NI Huafeng, YANG Guang, ZHANG Yanbing. ROP improvement technologies for large-cluster horizontal shale oil wells in the Changqing Oilfield[J]. Petroleum Drilling Techniques, 2021, 49(4): 29–33.
[6] 欧阳伟平,张冕,孙虎,等. 页岩油水平井压裂渗吸驱油数值模拟研究[J]. 石油钻探技术,2021,49(4):143–149. OUYANG Weiping, ZHANG Mian, SUN Hu, et al. Numerical simulation of oil displacement by fracturing imbibition in horizontal shale oil wells[J]. Petroleum Drilling Techniques, 2021, 49(4): 143–149.
[7] 王继伟,曲占庆,郭天魁,等. 考虑压裂液渗吸的压后压裂液返排的数值模拟[J]. 深圳大学学报(理工版),2023,40(1):56–65. WANG Jiwei, QU Zhanqing, GUO Tiankui, et al. Numerical simulation of post-fracturing flowback considering fracturing fluid imbibition[J]. Journal of Shenzhen University(Science & Engineering), 2023, 40(1): 56–65.
[8] 徐润滋,杨胜来,王吉涛,等. 高温高压下陆相致密油藏非稳态压裂液渗吸机理研究[J]. 油气地质与采收率,2023,30(3):94–103. XU Runzi, YANG Shenglai, WANG Jitao, et al. Study on unsteady imbibition mechanism of fracturing fluid in tight continental reservoirs under high temperature and high pressure[J]. Petroleum Geology and Recovery Efficiency, 2023, 30(3): 94–103.
[9] WANG Jinxun, DONG Mingzhe, YAO Jun. Calculation of relative permeability in reservoir engineering using an interacting triangular tube bundle model[J]. Particuology, 2012, 10(6): 710–721. doi: 10.1016/j.partic.2012.05.003
[10] LI Ran, CHEN Zhangxin, WU Keliu, et al. A fractal model for gas-water relative permeability curve in shale rocks[J]. Journal of Natural Gas Science and Engineering, 2020, 81: 103417. doi: 10.1016/j.jngse.2020.103417
[11] SU Yuliang, XU Jilong, WANG Wendong, et al. Relative permeability estimation of oil−water two-phase flow in shale reservoir[J]. Petroleum Science, 2022, 19(3): 1153–1164. doi: 10.1016/j.petsci.2021.12.024
[12] 何利,肖阳,孙宜成,等. 车21井区裂缝性油藏地质建模与工程设计一体化研究[J]. 特种油气藏,2021,28(5):23–29. HE Li, XIAO Yang, SUN Yicheng, et al. On integration of geological modeling and engineering design of fractured oil reservoirs in Well Block Che 21[J]. Special Oil & Gas Reservoirs, 2021, 28(5): 23–29.
[13] 孟勇,贾庆升,张潦源,等. 东营凹陷页岩油储层层间干扰及裂缝扩展规律研究[J]. 石油钻探技术,2021,49(4):130–138. MENG Yong, JIA Qingsheng, ZHANG Liaoyuan, et al. Research on interlayer interference and the fracture propagation law of shale oil reservoirs in the Dongying Sag[J]. Petroleum Drilling Techniques, 2021, 49(4): 130–138.
[14] 何吉祥,徐有杰,高阳,等. 裂缝性致密油藏多级压裂水平井试井模型[J]. 断块油气田,2021,28(2):241–246. HE Jixiang, XU Youjie, GAO Yang, et al. Well test model of multi-stage fractured horizontal well in fractured tight reservoirs[J]. Fault-Block Oil & Gas Field, 2021, 28(2): 241–246.
[15] 赵国翔,姚约东,王链,等. 页岩油藏微尺度流动特征及应力敏感性分析[J]. 断块油气田,2021,28(2):247–252. ZHAO Guoxiang, YAO Yuedong, WANG Lian, et al. Microscale transport behaviors of shale oil and stress sensitivity analysis[J]. Fault-Block Oil and Gas Field, 2021, 28(2): 247–252.
[16] 李江,陈先超,高平,等. 考虑应力敏感效应的裂缝性碳酸盐岩气井拟稳态产能预测方法[J]. 石油钻探技术,2021,49(3):111–116. doi: 10.11911/syztjs.2021032 LI Jiang, CHEN Xianchao, GAO Ping, et al. A pseudo-steady-state productivity prediction method for fractured carbonate gas wells considering stress-sensitivity effects[J]. Petroleum Drilling Techniques, 2021, 49(3): 111–116. doi: 10.11911/syztjs.2021032
[17] 李虹,于海洋,杨海烽,等. 裂缝性非均质致密储层自适应应力敏感性研究[J]. 石油钻探技术,2022,50(3):99–105. LI Hong, YU Haiyang, YANG Haifeng, et al. Adaptive stress sensitivity study of fractured heterogeneous tight reservoir[J]. Petroleum Drilling Techniques, 2022, 50(3): 99–105.
[18] LEE S H, LOUGH M F, JENSEN C L. Hierarchical modeling of flow in naturally fractured formations with multiple length scales[J]. Water Resources Research, 2001, 37(3): 443–455. doi: 10.1029/2000WR900340
[19] 刘礼军,姚军,孙海,等. 考虑启动压力梯度和应力敏感的页岩油井产能分析[J]. 石油钻探技术,2017,45(5):84–91. LIU Lijun, YAO Jun, SUN Hai, et al. The effect of threshold pressure gradient and stress sensitivity on shale oil reservoir productivity[J]. Petroleum Drilling Techniques, 2017, 45(5): 84–91.
[20] BAI Yuhu, LIU Lijun, FAN Weipeng, et al. Coupled compositional flow and geomechanics modeling of fractured shale oil reservoir with confined phase behavior[J]. Journal of Petroleum Science and Engineering, 2021, 196: 107608. doi: 10.1016/j.petrol.2020.107608
[21] 杨清立,杨正明,王一飞,等. 特低渗透油藏渗流理论研究[J]. 钻采工艺,2007,30(6):52–54. YANG Qingli, YANG Zhengming, WANG Yifei, et al. Study on flow theory in ultra-low permeability oil reservoir[J]. Drilling & Production Technology, 2007, 30(6): 52–54.
[22] LIU Lijun, HUANG Zhaoqin, YAO Jun, et al. Simulating two-phase flow and geomechanical deformation in fractured karst reservoirs based on a coupled hydro-mechanical model[J]. International Journal of Rock Mechanics and Mining Sciences, 2021, 137: 104543. doi: 10.1016/j.ijrmms.2020.104543
[23] 姚军,刘礼军,孙海,等. 复杂裂缝性致密油藏注水吞吐数值模拟及机制分析[J]. 中国石油大学学报(自然科学版),2019,43(5):108–117. YAO Jun, LIU Lijun, SUN Hai, et al. Numerical simulation and mechanism analysis of water huff and puff process in complex fractured tight oil reservoirs[J]. Journal of China University of Petroleum(Edition of Natural Science), 2019, 43(5): 108–117.
下载:
计量
- 文章访问数: 193
- HTML全文浏览量: 122
- PDF下载量: 72
