Calculation and Correction of Azimuth Errors Based on Finite Element Analysis
-
摘要:
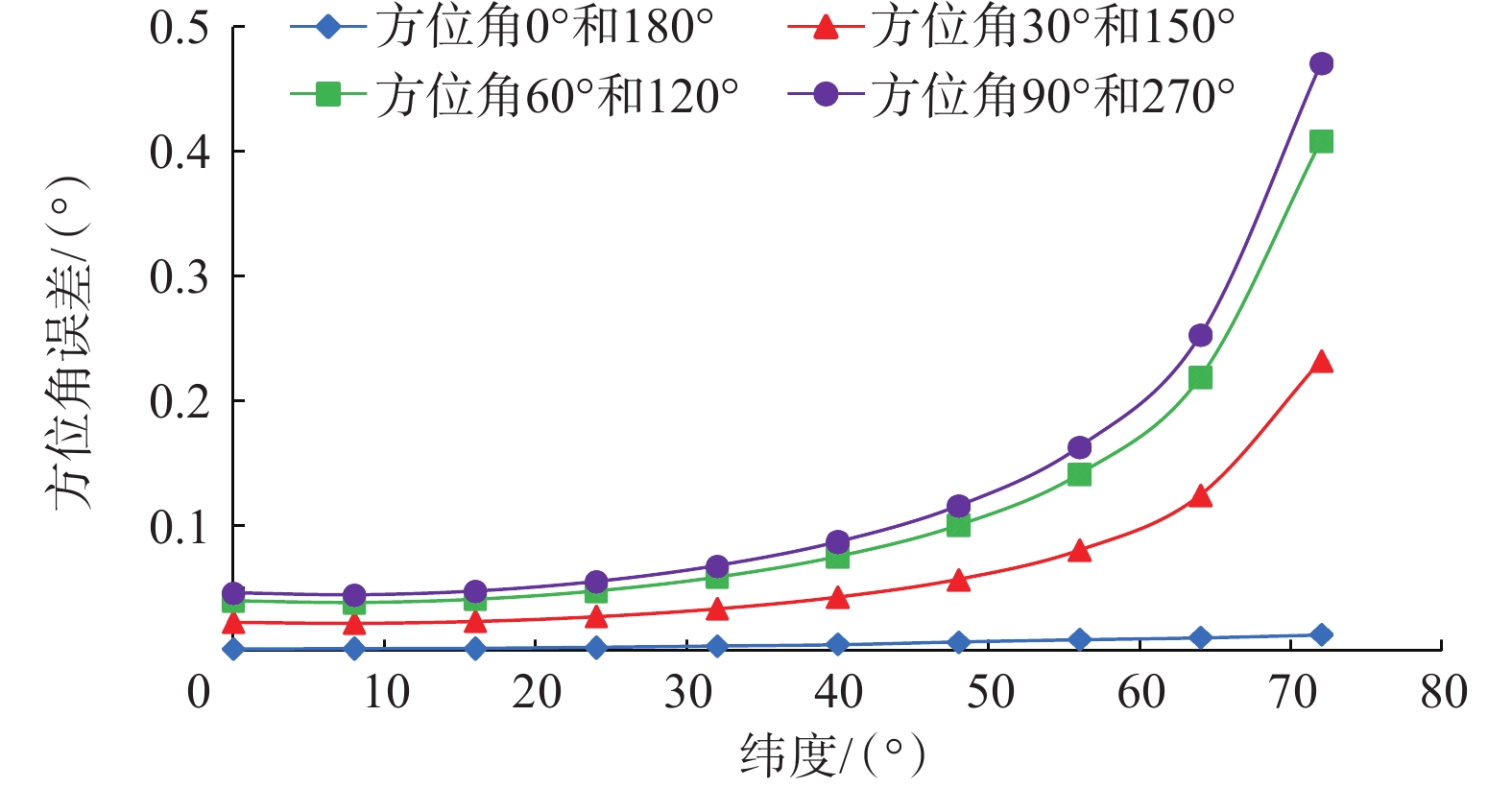
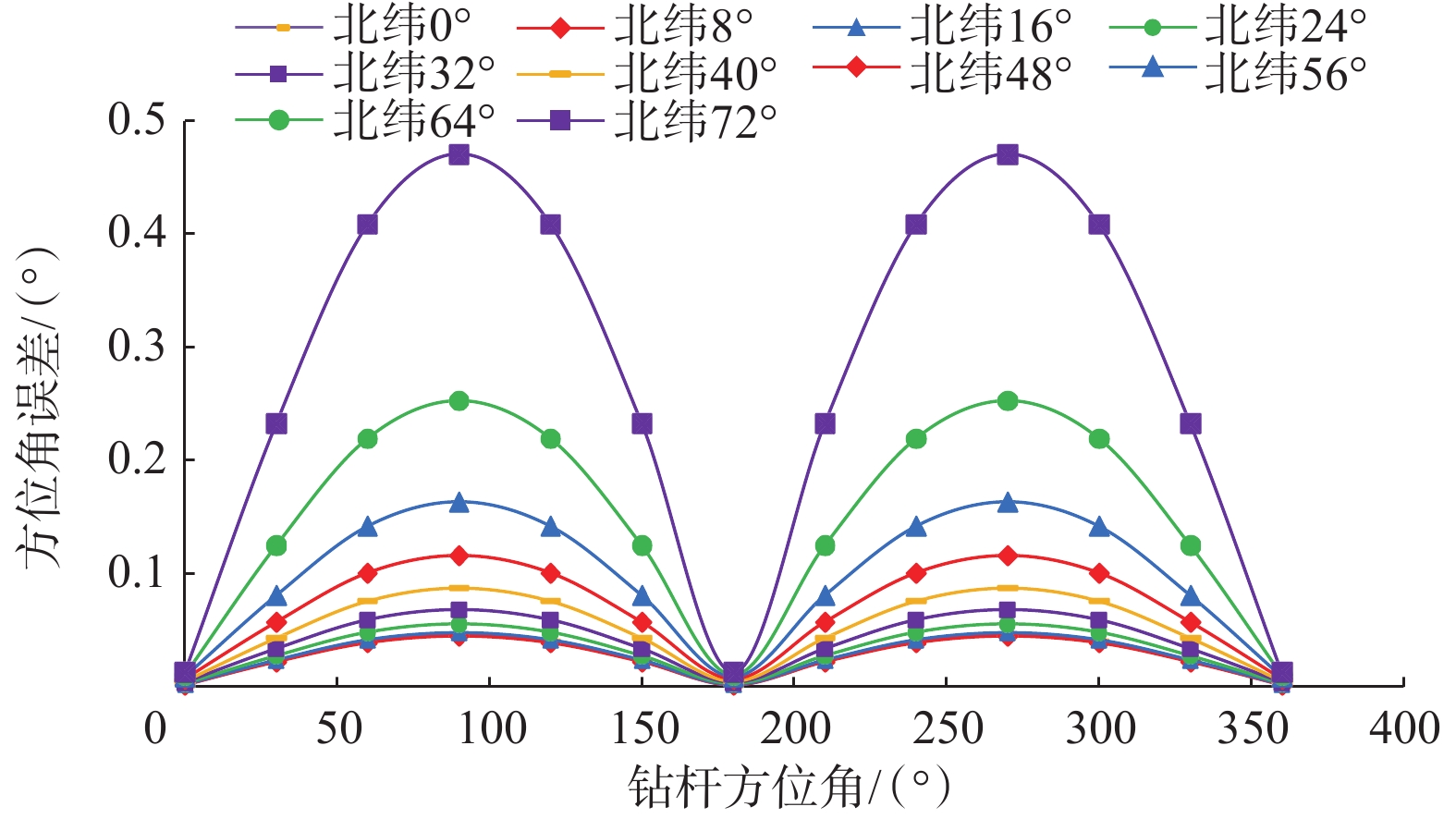
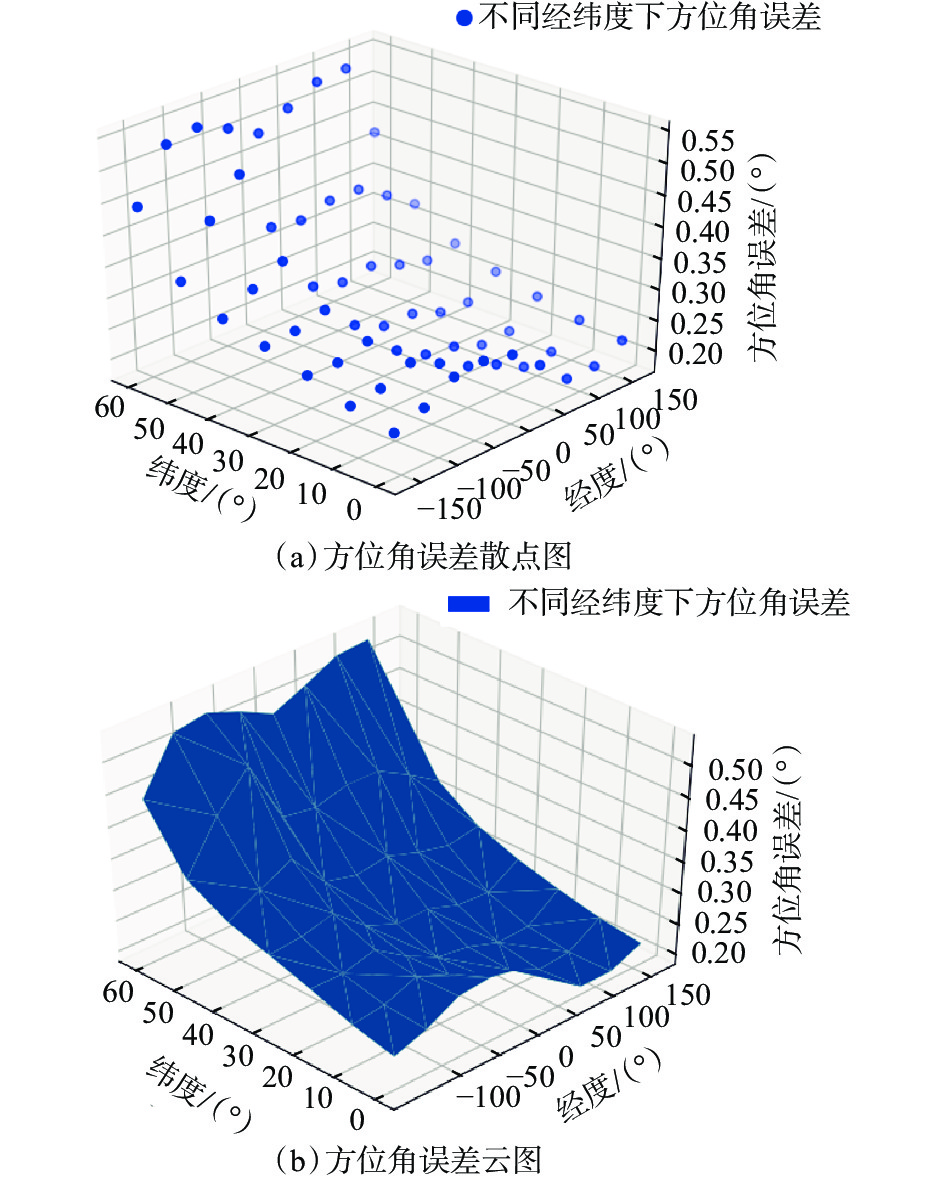
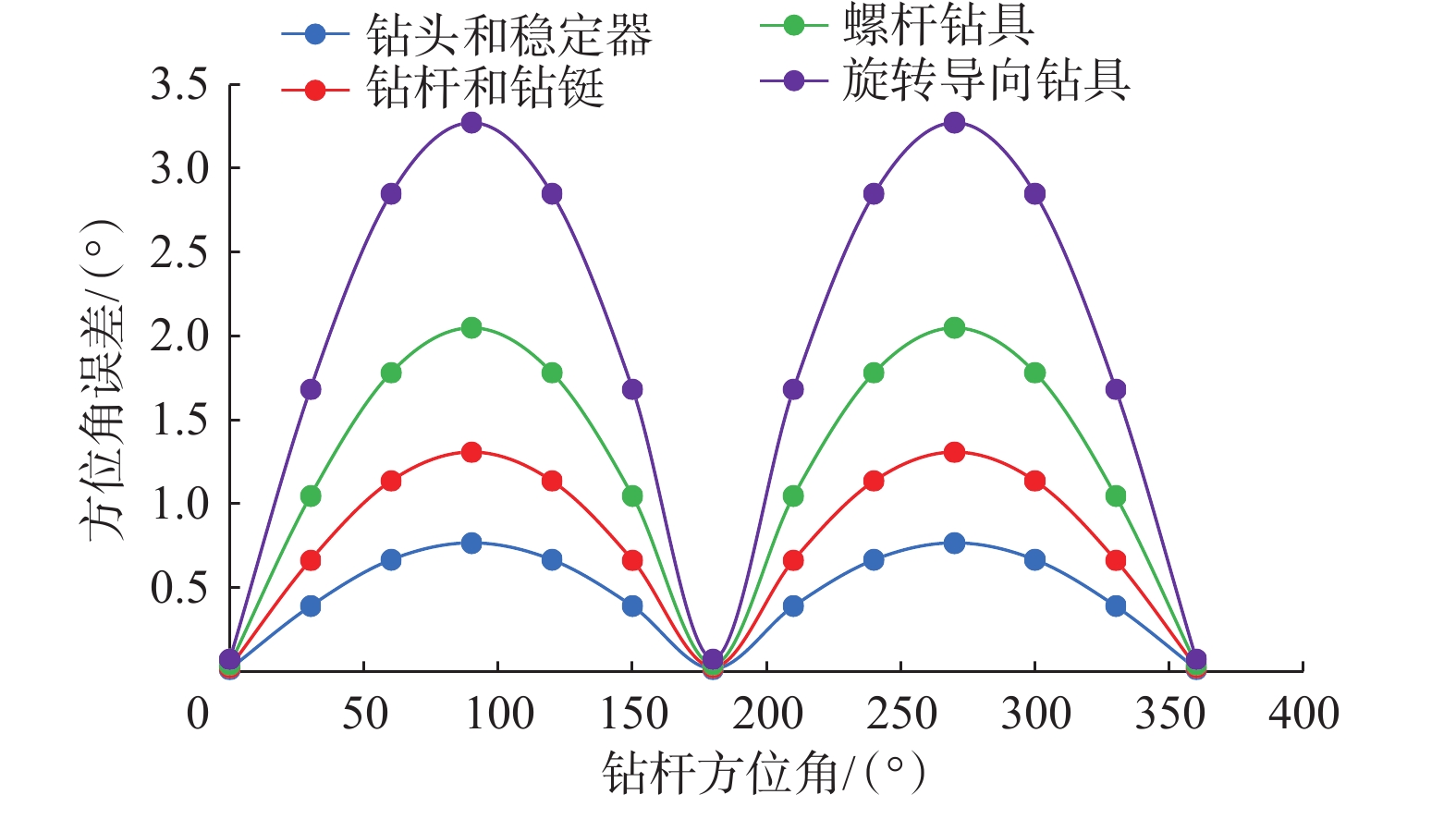
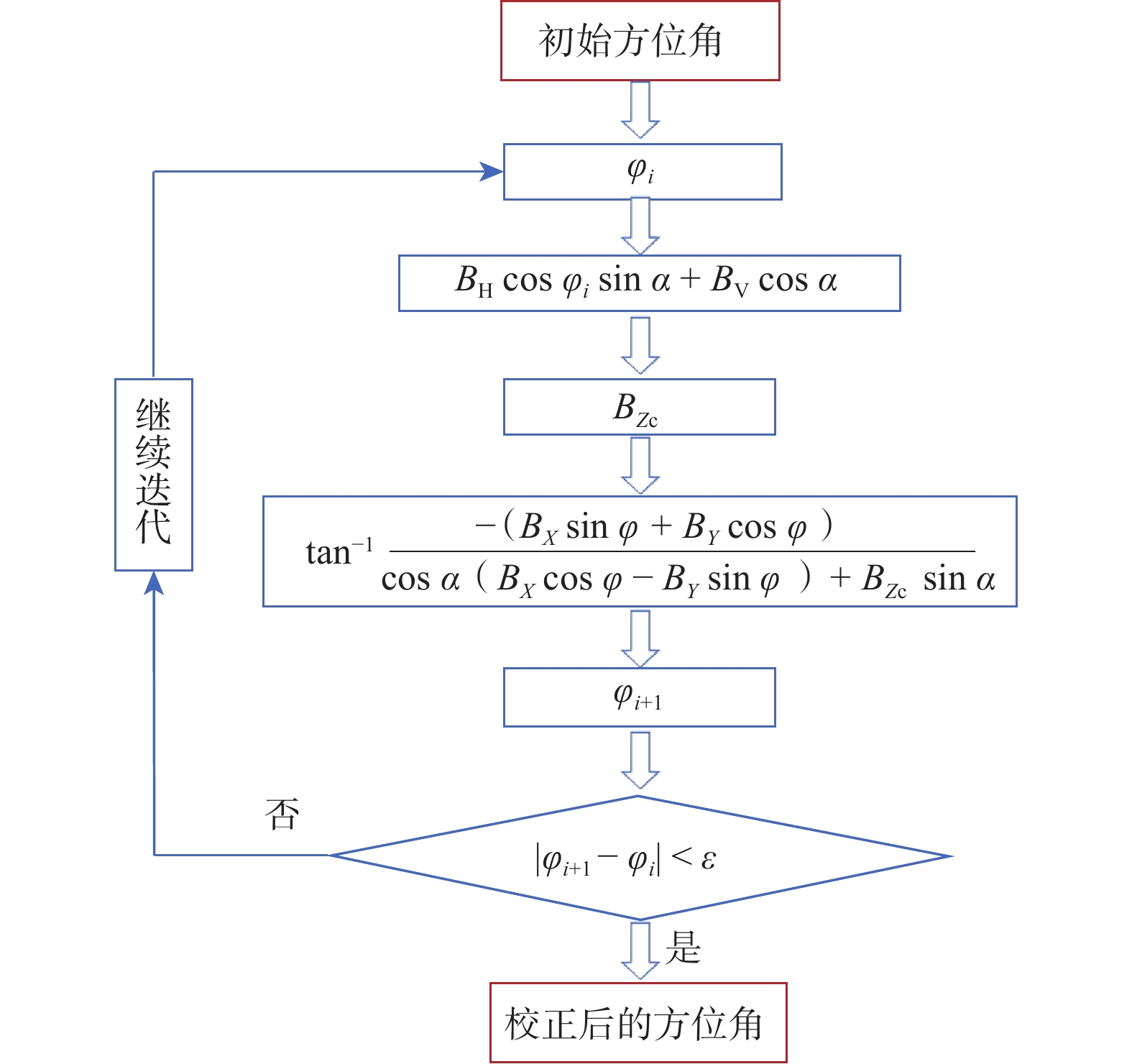
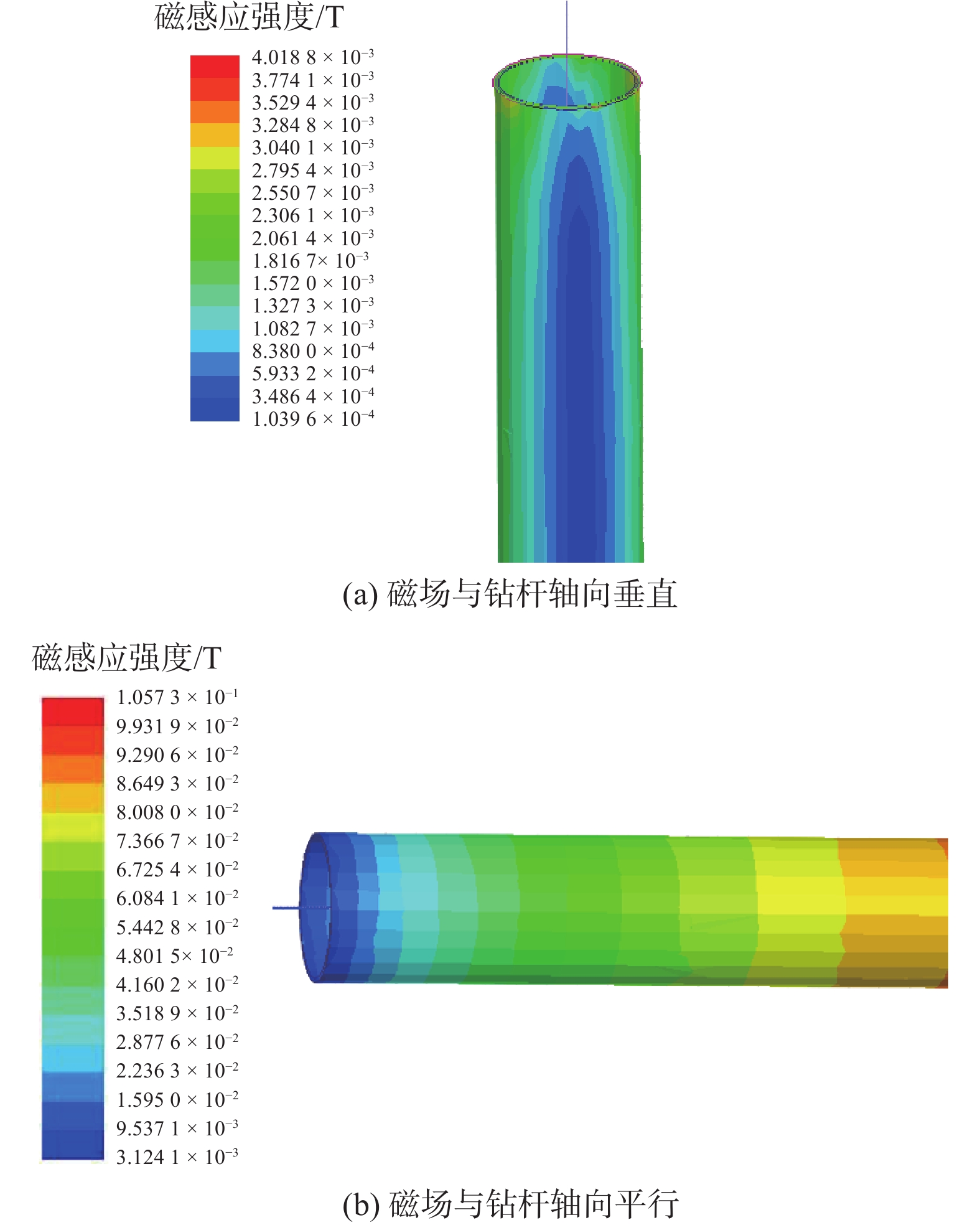
为了满足定向井磁方位的高精度需求，需要准确计算方位角测量误差和分析误差来源。采用有限元分析软件，模拟了钻具在地磁场中产生的干扰磁场的变化情况。通过钻杆内部三轴磁感应强度得出方位角误差的测量值，与钻杆真实方位对比得到了方位角误差。通过控制钻杆方位角、井斜角、底部钻具组合、无磁钻铤长度、外部磁场的大小和方向等因素，来观测、分析了方位角误差的变化规律。模拟试验得出，方位角误差在钻杆的南北方向上最小，在钻杆的东西方向上最大，并随着井斜角增大而增大，这种变化呈现出正弦的变化规律；经度、纬度也会对方位角的变化产生影响，方位角误差大体上随着维度增加而增大，主要取决于当地的总磁场和磁倾角大小。研究结果表明，方位角误差与测斜数据、底部钻具组合、经度、纬度和无磁钻铤长度有密切关系，可以通过有限元模拟准确估算出不同情况下方位角误差的大小，对方位角误差进行修正。模拟结果可为工程应用中无磁钻铤、底部钻具组合的选取和方位角误差修正提供参考数据和理论依据。
Abstract:The high-precision magnetic azimuth of directional wells requires the accurate calculation of azimuth measurement errors and analysis of error sources. In this study, the finite element analysis (FEA) software was used to simulate the change in the interference magnetic field generated by the drill tool in the geomagnetic field. The measured azimuth error was obtained through the triaxial magnetic induction intensity inside the drill pipe and was compared with the real azimuth of the drill pipe to get the azimuth error. Then, the variation laws of azimuth errors was observed and analyzed by controlling factors including the azimuth of the drill pipe, deviation angles, bottom hole assembly (BHA), non-magnetic drill collar length, and the size and direction of the external magnetic field. The simulation test showed that the azimuth error was the smallest in the north-south direction and the largest in the east-west direction of the drill pipe, and it increased with the rise in the deviation angles, which presented a sinusoidal variation law. Longitude and latitude would also affect the change in azimuth, and the azimuth error grew with the increase in dimensions in general and mainly depended on the local total magnetic field and magnetic inclination. The research results indicate that the azimuth error closely correlates with the inclinometer data, BHA, longitude, latitude, and the non-magnetic drill collar length, and it can be accurately estimated by finite element simulation under different conditions and then be corrected accordingly. The simulations can provide a reference and theoretical basis for the selection of the non-magnetic drill collar and BHA, and the correction of azimuth errors in engineering applications.
-
-
![]()
图 1 磁场垂直于和平行于钻杆轴向时的磁场强度分布
Figure 1. Magnetic field strength distribution when the magnetic field is perpendicular or parallel to the drill pipe axis
![]()
图 3 不同井斜角下钻具方位对磁测方位角误差的影响
Figure 3. Influence of drill tool azimuth on azimuth error of magnetic survey at different deviation angles
![]()
图 4 不同轨迹方位角下井斜角对磁测方位角误差的影响
Figure 4. Influence of deviation angles on azimuth error of magnetic survey under different azimuth of hole trajectories
![]()
图 6 不同纬度下方位角误差随钻杆方位的变化
Figure 6. Variations of azimuth error with drill pipe azimuth at different latitudes
![]()
图 7 不同经度和纬度下方位角误差的变化趋势
Figure 7. Variation trend of azimuth error under different longitudes and latitudes
![]()
图 10 4种不同钻具组合下钻具磁干扰引起方位角测量误差模拟结果
Figure 10. Simulation results of azimuth measurement errors caused by magnetic interference of four different BHAs
表 1 方位角误差模型采用的钻具数据
Table 1 Data of drill tools used in the azimuth error model
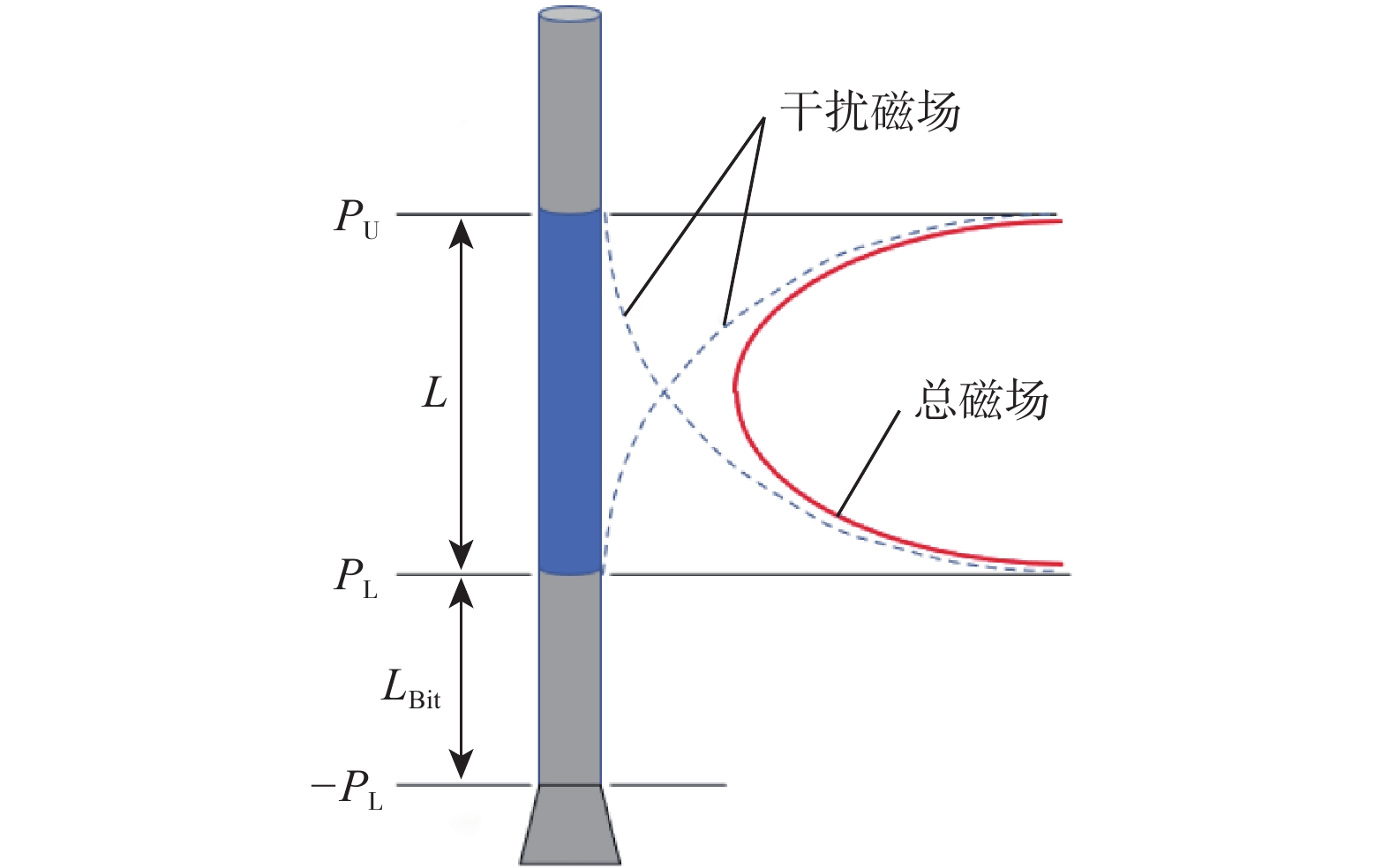
钻具或仪器 长度/mm 外径/mm PDC钻头 280 215.9 旋转导向工具 7 520 171.4 BCPM测井工具 4 940 171.4 集成井下测量仪 6 660 171.4 无磁钻铤 9 270 171.4 浮阀 680 165.1 加重钻杆 9 410 127.0 钻杆 9 410 127.0 
下载: 导出CSV
表 2 4种典型井底钻具组合对比
Table 2 Comparison of four typical BHAs
底部钻具组合 上部磁极强度/
μWb下部磁极强度/
μWb无磁钻铤距钻头的距离/m 钻头和稳定器 900 90 2 钻头和钻铤 900 300 10 钻头和螺杆钻具 900 1 000 10 钻头和旋转导向 900 3 000 10
下载: 导出CSV
-
[1] 詹世玉,吴银成,薛宏珍,等. 随钻测量磁干扰消除的研究[J]. 自动化与仪器仪表,2012(5):21–22. doi: 10.3969/j.issn.1001-9227.2012.05.010 ZHAN Shiyu, WU Yincheng, XUE Hongzhen, et al. Research on the magnetic interference elimination of MWD[J]. Automation & Instrumentation, 2012(5): 21–22. doi: 10.3969/j.issn.1001-9227.2012.05.010
[2] 毛建华,王清江. MWD磁干扰的分析判断方法探讨[J]. 钻采工艺,2008,31(3):129–130. doi: 10.3969/j.issn.1006-768X.2008.03.042 MAO Jianhua, WANG Qingjiang. Discussion on analysis and judgment method of MWD magnetic interference[J]. Drilling & Production Technology, 2008, 31(3): 129–130. doi: 10.3969/j.issn.1006-768X.2008.03.042
[3] 宋晓健,董晨曦. 磁性测斜工具中关于方位角校正的研究[J]. 石化技术,2015,22(9):181. doi: 10.3969/j.issn.1006-0235.2015.09.137 SONG Xiaojian, DONG Chenxi. Study on the correction of azimuth angle in magnetic measurement[J]. Petrochemical Industry Technology, 2015, 22(9): 181. doi: 10.3969/j.issn.1006-0235.2015.09.137
[4] 王小龙. 铁磁钻具引起测斜仪方位角误差的校正方法研究[J]. 地球物理学进展,2019,34(6):2521–2525. doi: 10.6038/pg2019CC0518 WANG Xiaolong. Nearly horizontal drill hole attitude measurement and aizmuth error correction caused by drilling tool[J]. Progress in Geophysics, 2019, 34(6): 2521–2525. doi: 10.6038/pg2019CC0518
[5] WILLIAMSON H S. Accuracy prediction for directional measurement while drilling[J]. SPE Drilling & Completion, 2000, 15(4): 221–233.
[6] 张苏,管志川,王建云,等. 邻井套管对井斜方位角的影响[J]. 石油钻探技术,2013,41(6):51–55. doi: 10.3969/j.issn.1001-0890.2013.06.010 ZHANG Su, GUAN Zhichuan, WANG Jianyun, et al. Impact of casing on adjacent well azimuth[J]. Petroleum Drilling Techniques, 2013, 41(6): 51–55. doi: 10.3969/j.issn.1001-0890.2013.06.010
[7] GRINDROD S J, WOLFF J M. Calculation of NMDC length required for various latitudes developed from field measurements of drill string magnetisation[R]. SPE 11382, 1983.
[8] 狄勤丰,芮子翔,周星,等. 带旋转导向工具的底部钻具组合横向振动特性研究[J]. 石油钻探技术,2021,49(6):8–16. doi: 10.11911/syztjs.2021059 DI Qinfeng, RUI Zixiang, ZHOU Xing, et al. Research on lateral vibration characteristics of bottom hole assembly with rotary steerable tool[J]. Petroleum Drilling Techniques, 2021, 49(6): 8–16. doi: 10.11911/syztjs.2021059
[9] 刘修善. 井眼轨迹精准定位技术[J]. 石油钻探技术,2017,45(5):8–12. LIU Xiushan. Precise positioning technique for wellbore trajectories[J]. Petroleum Drilling Techniques, 2017, 45(5): 8–12.
[10] 刘修善. 定向钻井轨道设计与轨迹计算的关键问题解析[J]. 石油钻探技术,2011,39(5):1–7. doi: 10.3969/j.issn.1001-0890.2011.05.001 LIU Xiushan. Analysis of the key issues involved in wellpath planning and trajectory calculation for directional drilling[J]. Petroleum Drilling Techniques, 2011, 39(5): 1–7. doi: 10.3969/j.issn.1001-0890.2011.05.001
[11] 王明哲,陆斌,于跃军. Sperry-Sun MWD轴向磁干扰的解决方法及应用[J]. 石油仪器,2008,22(2):47–49. WANG Mingzhe, LU Bin, YU Yuejun. Solution to the axial magnetic interference on Sperry-Sun MWD[J]. Petroleum Instruments, 2008, 22(2): 47–49.
[12] 许昊东,黄根炉,张然,等. 磁力随钻测量磁干扰校正方法研究[J]. 石油钻探技术,2014,42(2):102–106. XU Haodong, HUANG Genlu, ZHANG Ran, et al. Method of magnetic interference correction in survey with magnetic MWD[J]. Petroleum Drilling Techniques, 2014, 42(2): 102–106.
[13] 范光第,蒲文学,赵国山,等. 磁力随钻测斜仪轴向磁干扰校正方法[J]. 石油钻探技术,2017,45(4):121–126. FAN Guangdi, PU Wenxue, ZHAO Guoshan, et al. Correction methods for axial magnetic interference of the magnetic inclinometer while drilling[J]. Petroleum Drilling Techniques, 2017, 45(4): 121–126.
[14] 孟卓然. BHA轴向磁干扰对方位测量误差的影响: 基于人工磁场模拟方法[J]. 石油学报,2020,41(8):1011–1018. doi: 10.7623/syxb202008010 MENG Zhuoran. Effect of BHA axial magnetic interference on the azimuth measurement error: a simulation method based on artificial magnetic field[J]. Acta Petrolei Sinica, 2020, 41(8): 1011–1018. doi: 10.7623/syxb202008010
[15] 刘修善,王继平. 基于大地测量理论的井眼轨迹监测方法[J]. 石油钻探技术,2007,35(4):1–5. doi: 10.3969/j.issn.1001-0890.2007.04.001 LIU Xiushan, WANG Jiping. A method for monitoring wellbore trajectory based on the theory of geodesy[J]. Petroleum Drilling Techniques, 2007, 35(4): 1–5. doi: 10.3969/j.issn.1001-0890.2007.04.001
[16] 范光第,隋海东,黄根炉,等. 基于ANSYS的邻井套管磁干扰有限元仿真[J]. 石油机械,2013,41(8):1–4. doi: 10.3969/j.issn.1001-4578.2013.08.001 FAN Guangdi, SUI Haidong, HUANG Genlu, et al. ANSYS-based finite element simulation of magnetic disturbance of casing in adjacent well[J]. China Petroleum Machinery, 2013, 41(8): 1–4. doi: 10.3969/j.issn.1001-4578.2013.08.001
[17] FINLAY C C, MAUS S, BEGGAN C D, et al. International geomagnetic reference field: the eleventh generation[J]. Geophysical Journal International, 2010, 183(3): 1216–1230. doi: 10.1111/j.1365-246X.2010.04804.x
-
期刊类型引用(12)
1. 祝效华，李柯，李文哲，贺明敏，佘朝毅，谭宾. 万米深井上部大尺寸井眼钻柱动力学特性研究. 天然气工业. 2024(01): 49-57 . 
百度学术
2. 刘涛，何淼，张亚，陈鑫，阚正玉，王世鸣. 小井眼超深井井筒温度预测模型及降温方法研究. 钻采工艺. 2024(03): 65-72 . 百度学术
3. 刘忠飞，刘江峰，王孝亮，张耀晖，袁中涛，张子灏，李致宏. 深层环境下固井水泥水化放热特性及井周温度场演化规律研究. 钻采工艺. 2024(03): 161-169 . 百度学术
4. 宋先知，姚学喆，许争鸣，周蒙蒙，王庆辰. 超深井控温钻井隔热涂层参数影响机制研究. 石油钻探技术. 2024(02): 126-135 . 本站查看
5. 刘平江，和建勇，张晔，毕毅，张瑞华，杨谋. 高温深井温压耦合下流体性质对井筒压力的影响特性. 钻井液与完井液. 2024(03): 288-295 . 百度学术
6. 刘金璐，李军，柳贡慧，李辉，杨宏伟. 深水固井循环阶段井筒温度场预测模型研究. 石油钻探技术. 2024(04): 66-74 . 本站查看
7. 叶辉兵，张所邦，胡胜华，洪毅，刘航铭，陈维保，梁五宝，万宇航. 中深层地热井开采对套管与水泥环性能影响的研究. 钻探工程. 2024(05): 93-100 . 百度学术
8. 米晓军，刘东伟，杨保健，闫成双，关皓纶. 精细控压钻井技术研究及应用现状浅析. 中国石油和化工标准与质量. 2024(19): 186-189 . 百度学术
9. 龚巧龙. 莺-琼盆地海洋钻井井筒温度预测与分析. 阀门. 2024(10): 1165-1169 . 百度学术
10. 胡景宏，方博，王晓冬. 高含硫气藏地层温度场及硫沉积相态预测模型. 天然气工业. 2024(11): 92-100 . 百度学术
11. 柳军，简屹林，陈益丽，周鑫钟，梁爽，袁明健. 超深井射孔冲击振动模型及减振器振动抑制研究. 特种油气藏. 2024(05): 146-154 . 百度学术
12. 梁晓阳，赵聪，赵向阳，张亚洲，白园园，杨谋. 基于热管技术的钻井液地面降温系统研制. 石油机械. 2023(03): 24-32 . 百度学术
其他类型引用(2)
计量
- 文章访问数: 437
- HTML全文浏览量: 293
- PDF下载量: 49
- 被引次数: 14
